chat GPTで地域おこし小説【私は里山に住む女子大生】
美咲は、父が経営するふじの寺子屋で、子供たちに数学を教えていた。オンラインや学校、塾では教えることができない独自の方法で、コンパスと定規を使った数学を中心に教えていた。彼女の教え方は、子供たちにとって新鮮で刺激的であり、彼らは美咲の授業を楽しみにしていた。
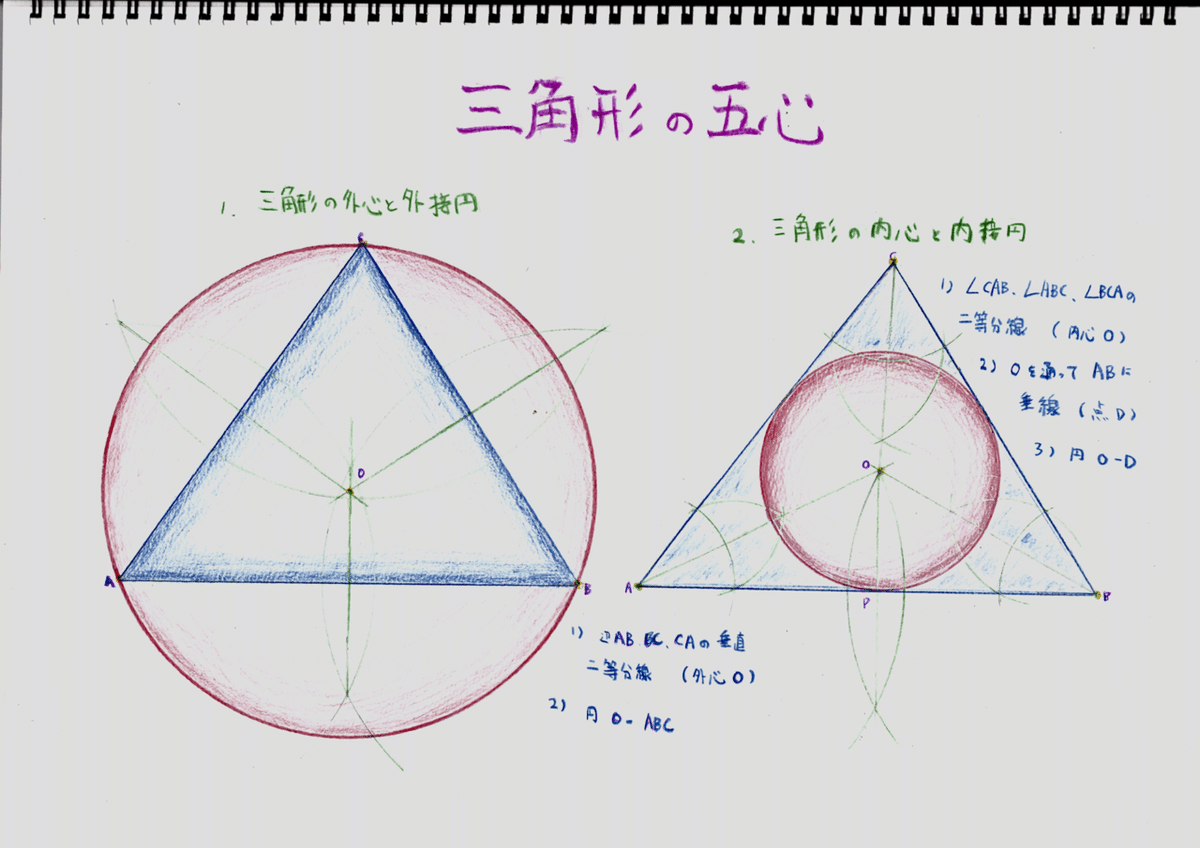
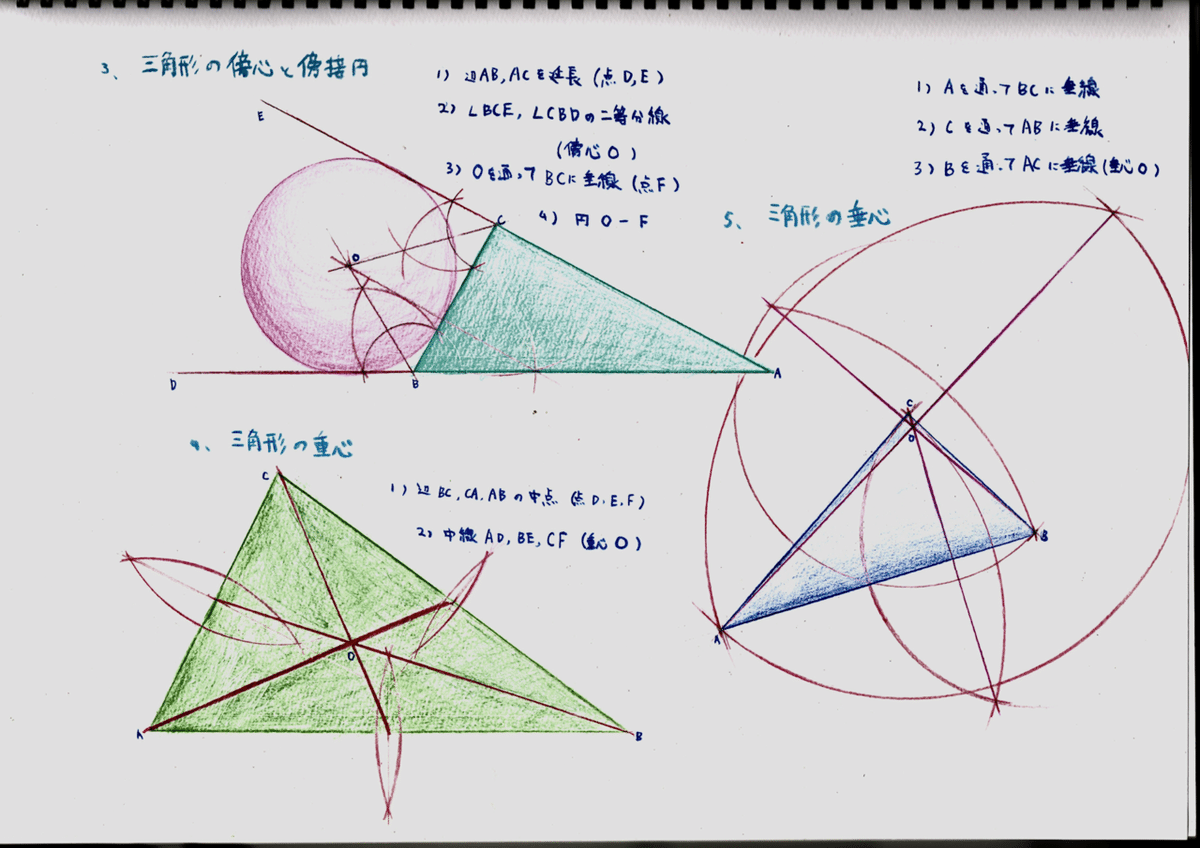
ある日、美咲は三角形の五心について教えることにした。五心とは、三角形に関連する重要な5つの中心点で、それぞれ重心、外心、内心、垂心、傍心と呼ばれている。これらの五心には興味深い性質がたくさんあり、美咲はそれらを子供たちに分かりやすく説明した。
重心は、三角形の各辺の中点を結んだ中線の交点であり、三角形の重心は重力のバランスがとれる点である。外心は、三角形の各辺の垂直二等分線の交点で、外接円の中心でもある。内心は、三角形の各角の角の二等分線の交点で、内接円の中心になる。各頂点から向かい合う辺におろした垂線の交点は垂心になる。1つの角の二等分線と残り2つの角の外角の二等分線の交点は傍心円の中心となる。
美咲は、これらの五心を実際にコンパスと定規を使って子供たちに説明しながら、彼らにも実践させた。子供たちは五心の神秘的な性質に興味津々で、美咲の授業に引き込まれていった。彼らは、数学の中にもリトリートのような楽しみがあることを実感し、数学がどれほど神秘的で魅力的なものであるかを学んだ。
美咲は、里山の寺子屋で子供たちに数学を教えることで、彼らに新しい世界を見せ、心と知性を豊かにする機会を与えていた。そして、美咲自身も、子供たちと共に学び成長していく喜びを感じていた。彼女は、子供たちが数学の中に隠された美しさや智慧を発見し、自分たちの人生に活かしていくことを願っていた。
寺子屋での授業が終わると、美咲は子供たちと一緒に里山を散策し、自然と触れ合う時間を楽しんだ。彼らは、山で見つけた形や模様が数学的な概念と関連していることに気づき、自然の中にも数学の美しさを見出すことができた。
Misaki taught mathematics to children at Fujino Juku, a temple school located on her father's rural land. Using her unique methods that couldn't be found in online classes, schools, or cram schools, she focused on teaching mathematics using a compass and ruler. Her teaching style was fresh and exciting for the children, who looked forward to her lessons.
One day, Misaki decided to teach the children about the five centers of a triangle. These centers are five important points related to a triangle, called the centroid, circumcenter, incenter, orthocenter, and excenter. These five centers have many interesting properties, which Misaki explained to the children in an easy-to-understand way.
The centroid is the point of intersection of the medians that connect the midpoint of each side of the triangle. The centroid is the balance point of the triangle, where the gravitational forces are balanced. The circumcenter is the intersection point of the perpendicular bisectors of each side of the triangle, and it is also the center of the circumcircle. The incenter is the intersection point of the angle bisectors of each angle of the triangle, and it is also the center of the incircle. The point of intersection of the altitudes dropped from each vertex to the opposite side is the orthocenter. The intersection point of one angle bisector and the bisectors of the exterior angles of the other two angles is the center of the excenter circle.
Misaki demonstrated the five centers using a compass and ruler, and let the children practice as well. The children were fascinated by the mysterious properties of the five centers and were drawn into Misaki's class. They realized that there is enjoyment in math, just like going on a retreat, and learned how fascinating and mysterious math can be.
By teaching math to children at the temple school on her father's rural land, Misaki gave them the opportunity to see a new world and enrich their hearts and minds. Misaki herself also felt the joy of learning and growing with the children. She hoped that the children would discover the beauty and wisdom hidden in math and apply it to their own lives.
After the class at the temple school ended, Misaki and the children went on a walk through the rural land, enjoying their time in nature. They noticed that the shapes and patterns they found in the mountains were related to mathematical concepts, and they were able to find the beauty of math in nature as well.
#CompassAndRuler #TriangleIncenter #数学 #教育 #自然 #田舎 #五心 #重心 #外心 #内心 #垂心 #傍心 #コンパス #定規 #美咲
#FujinoTerakoya #ふじの寺子屋 #数学教室
#UniqueMathematics #Math #Education #Nature #FiveCenters #Centroid #Circumcenter #Incenter #Orthocenter #Excenter #Compass #Misaki