【探究】sin15°

視点を変えながら，$${\sin15°}$$を考えよう。

後半の３つは直角三角形の定規があると楽しめる解法です。

➀　数学Ⅰ（余弦定理）

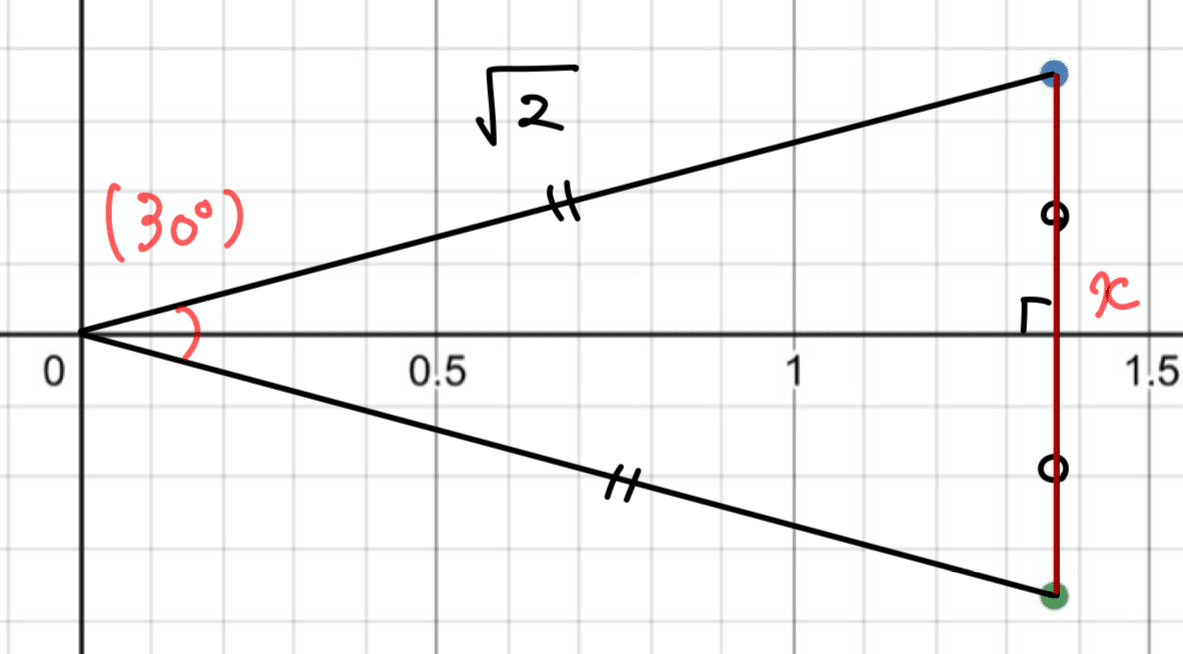
　頂角が30°の二等辺三角形を考える。２辺の長さを$${\sqrt{2}}$$，頂角の対辺の長さを$${x}$$とすると，余弦定理より

　　$${x^{2}=(\sqrt{2})^{2}+(\sqrt{2})^{2}-2\cdot\sqrt{2}\cdot\sqrt{2}\cdot\cos30°=4-2\sqrt{3}=(\sqrt{3}-1)^{2}}$$

　　$${x>0}$$なので，$${x=\sqrt{3}-1}$$

　ここで，頂角から底辺に垂線を下ろす。

　直角三角形の15°の対辺の長さが$${\frac{x}{2}=\frac{\sqrt{3}-1}{2}}$$なので

　　$${\sin15°=\frac{\sqrt{3}-1}{2\sqrt{2}}=\frac{\sqrt{6}-\sqrt{2}}{4}}$$

②　数学Ⅱ（加法定理）

　　$${\sin(\alpha-\beta)=\sin\alpha\cos\beta -\cos\alpha\sin\beta}$$ より

　　$${\sin(45°-30°)=\sin45°\cos30°-\cos45°\sin30°}$$

　　　　　 $${\sin15°=\frac{\sqrt{2}}{2}\cdot\frac{\sqrt{3}}{2} -\frac{\sqrt{2}}{2}\cdot\frac{1}{2}=\frac{\sqrt{6}-\sqrt{2}}{4}}$$

③　数学Ⅱ（２倍角の公式）

　　$${\sin^{2}\theta=\frac{1}{2}(1-\cos 2\theta)}$$ より

　　$${\sin^{2}15°=\frac{1}{2}(1-\cos 30°)=\frac{1}{2}(1-\frac{\sqrt{3}}{2})=\frac{2-\sqrt{3}}{4}}$$

　　　　　　$${=\frac{4-2\sqrt{3}}{8}=\frac{(\sqrt{3}-1)^{2}}{8}}$$

 　　$${\sin15°=\frac{\sqrt{3}-1}{\sqrt{8}}=\frac{\sqrt{6}-\sqrt{2}}{4}}$$

④　中学数学（三平方の定理）

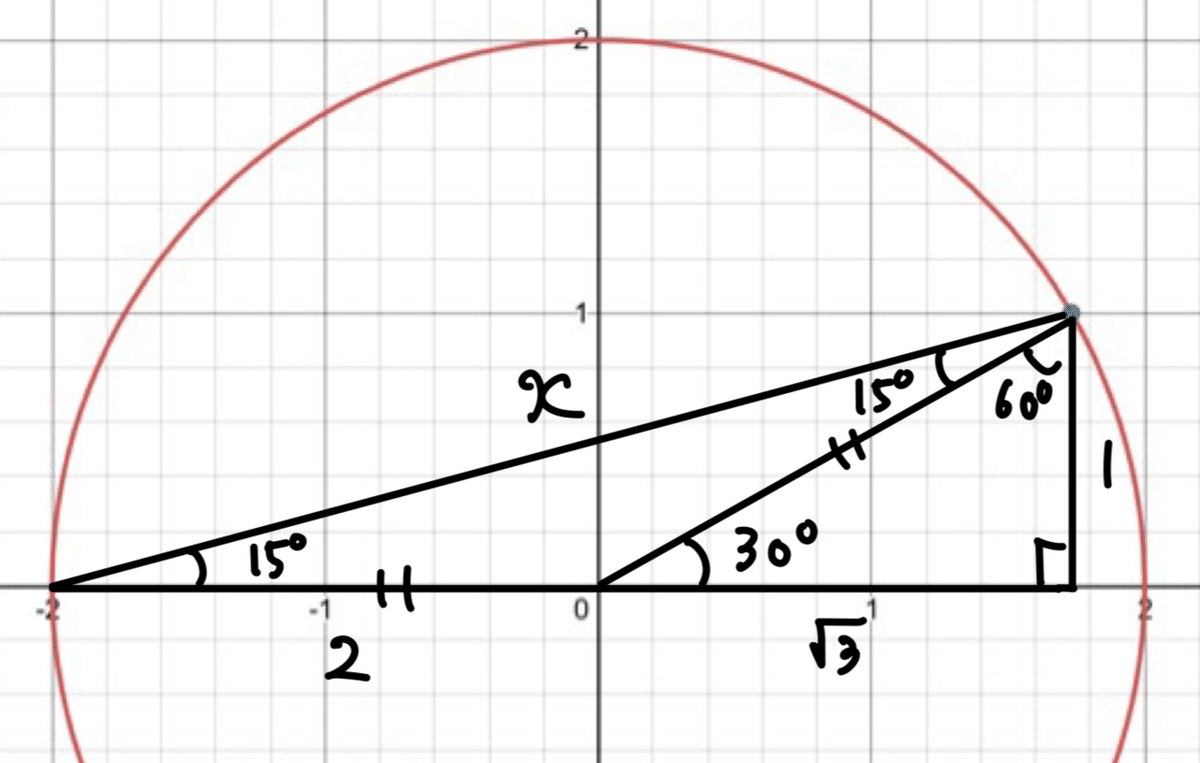
　図で，三平方の定理より

　　$${x^{2}=1^{2}+(2+\sqrt{3})^{2}=8+4\sqrt{3}=8+2\sqrt{12}=(\sqrt{6}+\sqrt{2})^{2}}$$

　　$${x>0}$$なので，$${x=\sqrt{6}+\sqrt{2}}$$

　よって，$${\sin15°=\frac{1}{x}=\frac{1}{\sqrt{6}+\sqrt{2}}=\frac{\sqrt{6}-\sqrt{2}}{4}}$$

⑤　数学ⅠA（トレミーの定理～正弦定理）

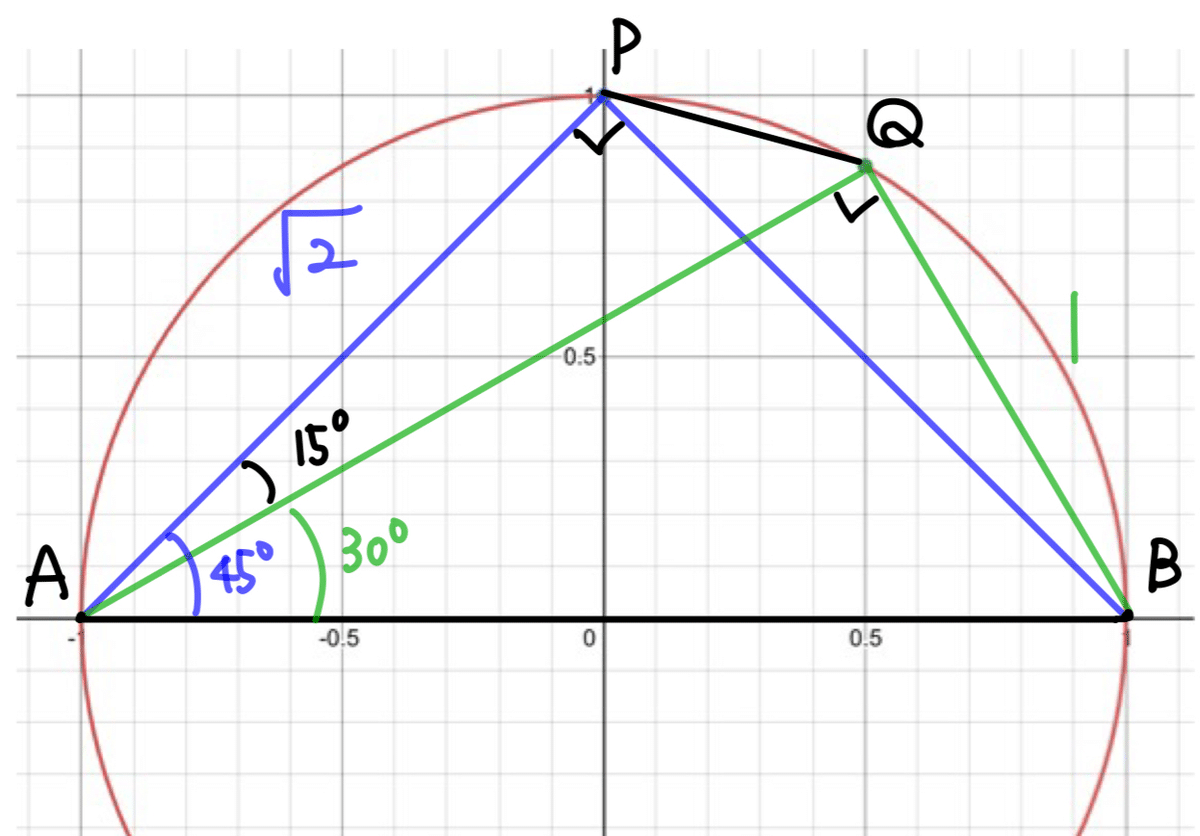
　斜辺の長さが２となる直角三角形を考える。

　斜辺ABに対して，直角となる２点Ｐ，Ｑが同一円周上にあるので，ＰＱ$${=x}$$として，トレミーの定理より

　　$${1\cdot\sqrt{2}+2x=\sqrt{2}\cdot\sqrt{3}}$$

　　　　$${x=\frac{\sqrt{6}-\sqrt{2}}{2}}$$

　△APQで，正弦定理より

　　$${\frac{x}{\sin15°}=2}$$

　　$${\sin15°=\frac{x}{2}=\frac{\sqrt{6}-\sqrt{2}}{4}}$$

⑥　数学Ⅰ（正弦定理）

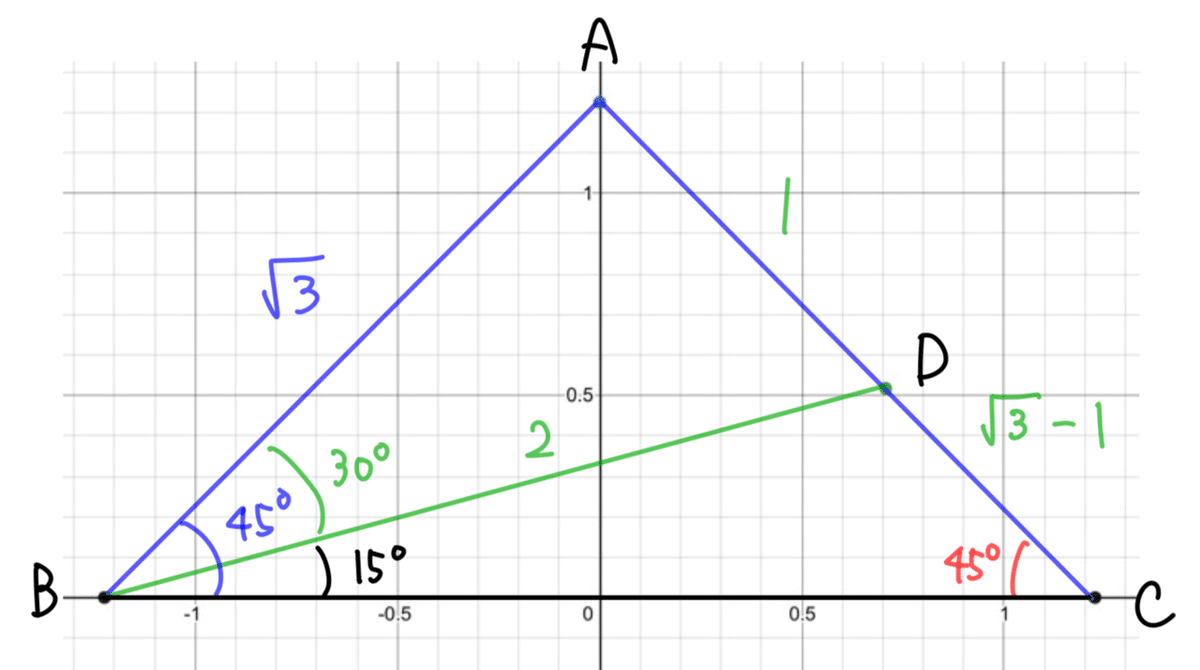
　△BCDで，正弦定理より

　　$${\frac{\sqrt{3}-1}{\sin15°}=\frac{2}{\sin45°}}$$

　　$${\sin15°=(\sqrt{3}-1)\cdot\sin45°\cdot\frac{1}{2}=\frac{\sqrt{6}-\sqrt{2}}{4}}$$