眠り姫問題の簡易説明 -(日本語&English)
眠り姫問題という”確率”を扱った思考実験があります。問題自体は小学生でも分かるくらいにシンプルなのに、今だに決定的な答えのないとても面白い問題です。要点をかいつまんで言うと下記のようなものです。
問題
実験の参加者である眠り姫は、実験の内容を全て説明され、一日経過後、特殊な眠り薬を投与され日曜日に眠りにつく。
眠り姫が眠っている間に一度だけ公正なコインが投げられる。
コインが表であった場合、眠り姫は月曜日に目覚めさせられ、質問されたのち、再び薬を投与され眠りにつく。
コインが裏であった場合、眠り姫は月曜日に目覚めさせられ、質問されたのち、再び薬を投与され眠りにつく。そして翌日の火曜日にも目覚めさせられ、質問されたのち、再び薬を投与され眠りにつく。
この時投与される薬は一日の記憶を完全に忘却する記憶消去薬で、次に目覚めさせられるまで絶対に目覚めないという作用がある。 眠り姫が目覚め質問を受ける際、その日が何日であるか、以前に目覚めたことがあるかどうかは決して知ることができないとする。
起こされた時にされる質問とは「コインが表だった確率は幾らか?」というものである。
どちらの場合でも、水曜日になれば眠り姫は目覚めさせられる。水曜日は質問を行わず、実験はそこで終了する。
引用元:https://ja.wikipedia.org/wiki/%E7%9C%A0%E3%82%8A%E5%A7%AB%E5%95%8F%E9%A1%8C
…
説明
眠り姫問題の要点は『公正なコイン』は表と裏の出る比率は1:1と等しいはずなのに、目覚めた眠り姫にとってはその観察比率が1:2と異なることです。
この思考実験で問題とされるのは、目覚めた眠り姫にとっての公正なコインで『表』が出た確率ですが、これを1/2とする見方と1/3とする見方に大きく人々の意見は分かれます。
眠り姫にとっての『表』の確率を1/2とするか1/3するかの違いは、目覚めた眠り姫の持つ観察比率の違いをどう解釈するかによって決まります。
その解釈の違いを考えるには以下のように確率の捉え方を整理する必要があります。
…
主観確率Pの構成要素を以下の3つと考えます。
A:『確率的構造体』。その構造に由来する固有確率を持つ。そのすべての根元事象の頻度が均等な時これは公正であり、そうでなければ不公正な構造体となる。公正な構造体の例は歪みの無いコインや均一なサイコロなどであり、不公正な例は歪んだコインや細工のされたサイコロなどが考えられる。確率的事象の対象となるすべてのものは確率的構造体と見做せ、それは天気や出来事、人の意思や行動など有形無形を問わない。
B:『行為主体』。Aを動的状態にしその固有確率を事象化する。サイコロやコインの様にその状態が静止して一定に定まるものは、Bがそれを新たに動的状態にしなければその固有確率は事象化しない。また、天気の様にAが常に動的状態にある場合Bは必要ないが、それは無作為で公正なBとして扱うことができる。行為主体はその事象化頻度をもち、それがAの事象化に際しその固有確率に影響を与えない場合それは公正であると言え、そうでなければ不公正な行為主体となる。不公正な行為主体の例はギャンブルで公正なサイコロやカードでトリックを使ってイカサマをするディーラーなど。
C:『観察主体』。Bによって事象化したAの固有確率を観察する主体。Cは観察機会と、観察機会ごとの観察量をもつ。確率とは『不明の対象』の確からしさや頻度を扱う概念であるため、確率は『観察』によってのみ事象として成立する。観察主体はその観察比率を持ち、観察に際してBによって事象化したAの結果に影響を与えない場合は公正であり、そうでなければ不公正な観察主体となる。不公正な観察主体とはその観察に条件や偏りをもちその観察比率が均等でない場合などである。例えば公正なコインを公正に投げた時、その観察者は目を瞑っており結果が『表』の時にしか目を開けないという条件がある場合、その観察者は不公正な観察者となる。
…
P: 行為主体によって事象化された、確率的構造体の固有確率を、観察主体が観察した時の、観察主体の確率的構造体に対する主観確率。
P = A x B x C
0 ≦ P ≦ 1
…
初めに述べたように眠り姫問題は、表と裏が同等の固有確率を持つ公正なコインに対し、観察主体(眠り姫)の観察比率が異なる事にあります。この観察比率の違いをどう解釈するかが、眠り姫にとってコインが表の主観確率を1/2とするか1/3とするかの違いとなります。
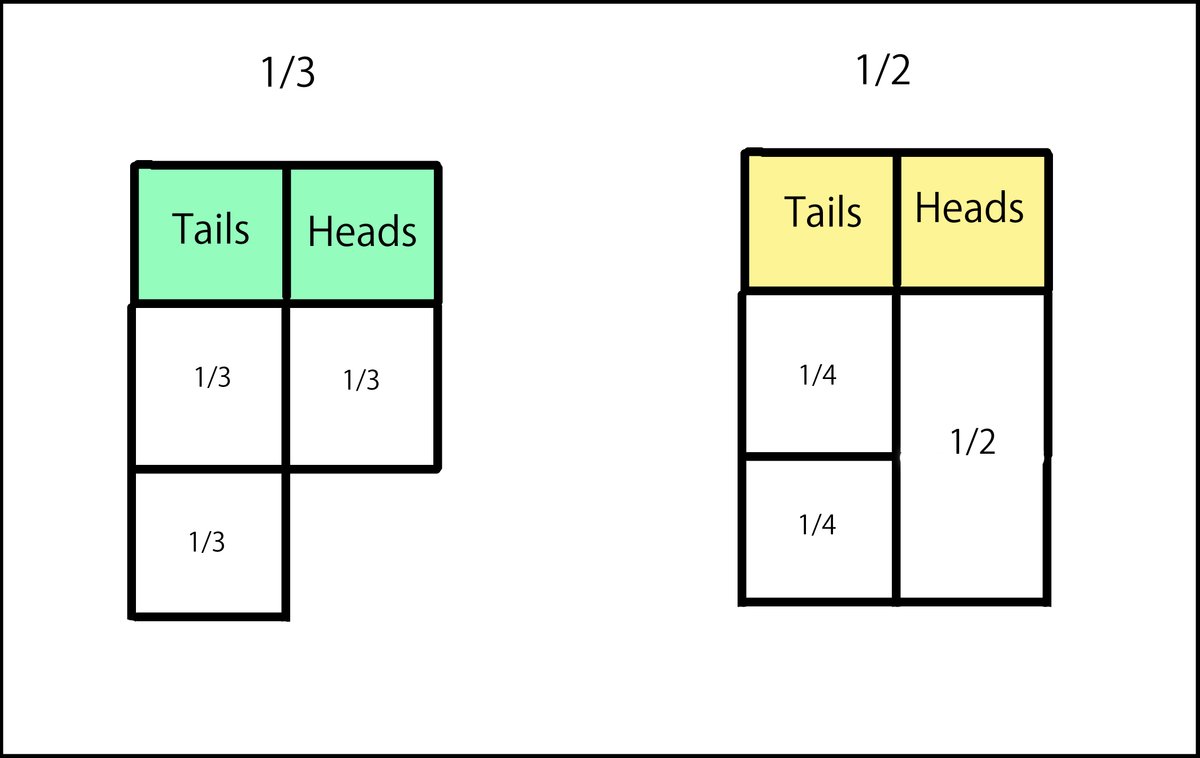
下記の図はどちらも”表(Heads)と裏 (Tails)”の観察比率(機会)が1:2の関係を現しています。
眠り姫にとって表が出る主観確率を1/3とする見方は、この観察比率の差を”表と裏”の観察機会ごとの観察量は公正だが、全体的な観察量が(表:裏=1:2)と異なっているという解釈です。
一方、1/2であるとする見方は表と裏の全体的な観察量は公正だが、観察機会ごとの観察量が(表:裏=2:1)となるという解釈です。
眠り姫の主観確率を1/3とする見方は、表と裏の観察機会ごとの観察量(確率量)は等しい為、行為主体によるコインの事象化頻度は公正と言えます。そしてこれは観察比率の違いを全体的な観察量の違いとする見方であり、観察主体の不公正を意味します。
一方、『表』の主観確率を1/2とする見方は、コインの表と裏の全体の観察量は公正だが、観察機会ごとの観察量が異なるという見方です。この解釈では一度の『表』の観察量が、二度の『裏』の観察量と等しくなります。これは公正なコインの表と裏の事象化頻度が異なっていると言う見方である為、行為主体の不公正を意味します。
要するに、眠り姫の主観確率を1/3とする見方は観察主体(眠り姫)の不公正を意味し、1/2とする見方は行為主体(実験者)の不公正を意味します。
…
そうなると問題はそのどちらの見方が妥当なのかになりますが、この眠り姫問題の設定ではコインの公正性は明言されていますが、実験者(行為主体)と眠り姫(観察主体)の公正性は明言されていません。そのため、この思考実験の設定では行為主体を不公正であるとして眠り姫の主観確率を1/2とする解釈も、観察者を不公正としてこれを1/3とする解釈も可能と言えます。
ですが、この実験の主旨は、条件付きの睡眠と目覚めを課された眠り姫の主観確率とその変化です。その問題の答えが、行為主体の不公正を理由に1/2と言うのは問題の主旨から言えば妥当性があるとは言い難く思えます。これは具体的に言えば、実験者がコイン投げでイカサマをしたから眠り姫のコインの主観確率は1/2であるという事です。実験者の公正性が保証されない限り眠り姫はそれを否定することができません。
よって、この問題の条件設定は眠り姫の主観確率を決定するには不十分であると考えられます。その妥当な修正としては、実験の設定の『公正なコイン』に加えて『実験者は公正にコインを投げる』という一文を追加することが考えられます。その場合観察比率の違いは観察主体の不公正と見なすことができ、目覚めた眠り姫にとってコインが表の主観確率は1/3と決定することができます。
あるいはこの問題設定を維持するならば、眠り姫の返答を下記のような質問で始める必要があります。
…
*実験者は眠り姫を起こして質問をした。
実験者:あなたが眠っている間に私が投げたこのコインで、『表』が出た確率は、あなたにとってどの程度ですか?
眠り姫:それを答える為には、あなたに一つ質問をする必要があります。
実験者:何でしょう?
眠り姫:あなたはその公正なコインを公正に投げましたか?もしそうであれば私にとってそのコインで表が出た確率は1/3です。
実験者:その理由は?
眠り姫:公正なコインが内在的に有する”表”が出る固有確率は常に1/2で変わることはありません。睡眠前には私にとってもそれは1/2でした。しかし実験の睡眠と目覚めの条件により、目覚めた私の『表と裏』の観察比率は『表:裏 = 1:2』になりました。ですがその解釈には二通りあります。
一つは(月曜:表)の確率を1/2、(月曜:裏)の確率を1/4、(火曜:裏)の確率を1/4とする見方です。この場合私にとってコインが表の確率は1/2です。しかし一度の『表』の観察機会の観察量が二つの裏の観察機会と等しくなるため、コインの事象化比率は不公正となります。これはあなたのコイン投げが不公正だったことを意味します。
もしあなたが公正にコインを投げたならば、『表と裏』の観察機会ごとの観察量(確率量)は等しくなります。コインが公正であり、あなたがそれを公正に投げたならば、この観察比率の違いは私の観察者としての公正性が失われたと解釈せねばなりません。表:裏の観察比率が1:2であり、観察機会ごとの確率が等しいならば、(月曜:表)、(月曜:裏)、(火曜:裏)の確率は全て等しく1/3となります。
よって、あなたが公正にコインを投げたのなら、私にとってコインが表の主観確率は1/3です。
…
この思考実験から学べることは、私たちが確率の概念をかなり曖昧で大雑把に扱っているということです。
具体的には、『公正なコイン』の問題を考えた場合、私たちはすぐに表と裏の確率がそれぞれ1/2だろうと考えがちです。しかし、確率的な事象が成立するには、常にコイン(確率的構造体)、コインを投げる人(行為主体)、コイン投げの結果を観察する人(観察主体)の三つが存在し、これら全てが公正でも不公正でもあり得るということをこの問題は示しています。
確率的構造体や行為者が不公正であることは、私たちは経験上よく知っています。たとえば、歪んだコインやイカサマをする人などがその例です。しかし、観察者の不公正性はあまり意識されることがありません。特に観察者が自分自身である場合、人はその公正性を疑わない傾向があると言えます。
人は自分の視点からしか世界を見ることができないため、公正な観察者であるという無条件の信念は、誰もが自然に持つものかもしれません。
自分自身が世界の観察者として公正であるという無条件の信念は、主観確率を扱う『眠り姫問題』においてもっとも重要で興味深い点です。
さらに面白いことは、この自らの公正性への無条件の信念は、同一性問題としても考えることができるということです。
確率と同一性
眠り姫の観察者としての公正性が実験の『睡眠と目覚め』によって失われることを、眠り姫の立場から見るとこの問題は眠り姫の同一性の問題とも見ることができます。
『表と裏』が同等の固有確率を持つコインが、公正な事象化頻度で事象化したにもかかわらず、目覚めた眠り姫にとって『表』が出る主観確率が1/3に変化している場合、眠り姫はそれを自らの観察者としての公正性が失われたと解釈せざるを得ません。
公正なコインの事象化頻度が1:1であることが事実言える場合、その結果に対して1:2の観察比率を持つためには、どのような視点、立場、位置、あるいは時空から観察することが必要なのか。これを眠り姫の立場から考えると、観察者としての今の自分は一体どんな時空のどのような位置にいるのか、私は睡眠前の私と同一の存在と言えるのかという問いに変わります。
観察者としての公正性を失った眠り姫は、少なくとも実験で使われたコインに対する観察主体としての同一性を失っているといえ、そして眠り姫は1:1で事象する対象を1:2の比率で観察することが可能なイレギュラーな『時空』に位置すると言えます。
眠り姫の同一性問題としてこの問題を考える時に『位置と時空』が問題になるのはこの思考実験が二つの確率で構成されている為です。その一つはコイン投げの表と裏の二択であり、もう一つは曜日決定の月曜か火曜の二択です。
コインの表と裏は1次元の空間的な選択であり、曜日決定は1次元の時間的選択です。そのため目覚めた眠り姫に与えられる可能な状態はそれらの組み合わせである、(表・月曜日)、(裏・月曜日)、(裏・火曜日)という(空間・時間)の2次元を組み合わせた根元事象になります。その結果として眠り姫は、空間(一次元)と時間(一次元)の組合せ事象を網羅的に俯瞰できる3次元的位置に視点を持つ事になります。
私はどこにいるのか、私は眠る前の自分と同一な存在かというのが、眠り姫の視点で見た時のこの問題の印象と感覚です。眠り姫がされる質問は『あなたにとってコインが表の確率はどの程度ですか?』というものですが、この質問の本質は眠り姫の同一性が維持されているのかを聞いているとも言えます。なぜならもし眠り姫が観察者としての同一性を維持しているならコインが表の確率は1/2のままであり、そうでなければ1/3だからです。
眠り姫問題は一つの側面から見れば確率の問題であり、別の面から見れば同一性の問題とも見ることが可能な問題です。確率と同一性はどちらも確からしさと度合いを扱いますが、眠り姫問題はその二つの密接な関係性を示している点でも非常に面白い問題と言えます。
…
23.05.13th
23.05.14th
23.05.18th
Sleeping Beauty Problem brief explanation - Eng
There is a thought experiment called the "Sleeping Beauty problem" that deals with "probability." Despite its simplicity, the problem remains intriguing as it lacks a definitive answer. The key points can be summarized as follows:
The problem:
The participant, known as Sleeping Beauty, is fully informed about the experiment and takes a drug that puts her to sleep on Sunday.
While Sleeping Beauty is asleep, a fair coin is flipped once.
If the coin lands Heads, Sleeping Beauty is awakened on Monday, asked a question, given the drug again, and put back to sleep.
If the coin lands Tails, Sleeping Beauty is awakened on Monday, asked a question, given the drug again, put back to sleep, and then awakened again on Tuesday, asked the same question, given the drug again, and put back to sleep.
The drug administered induces complete memory loss for the day, ensuring that Sleeping Beauty will never awaken until the next time.
When Sleeping Beauty is awakened and questioned, she can never know which day it is or if she has been awakened before.
The question asked upon awakening is, "What is your credence now for the proposition that the coin landed heads?"
In either case, Sleeping Beauty is always awakened on Wednesday, and the experiment ends without further questioning.
Understanding the problem:
The key point of this problem is the discrepancy between the expected 1:1 ratio for Heads and Tails of a fair coin and the awakened Sleeping Beauty's observation ratio of the coin which is 1:2 for Heads:Tails.
In this thought experiment, the crucial issue is determining the probability of "Heads" for the fair coin from the perspective of the awakened Sleeping Beauty. There is a significant divide in opinions between those who interpret it as 1/2 and those who interpret it as 1/3.
The difference in interpreting the probability of "Heads" for the Sleeping Beauty depends on how one interprets the disparity in observed ratios for the awakened Sleeping Beauty. To explore this difference, it is necessary to clarify our understanding of probability.
…
We can consider the three elements that make up subjective probability P
A : "Probabilistic structure." It has an inherent probability derived from its structure. When the frequency of all elementary events is equal, it is fair, otherwise it is an unfair structure. Examples of fair structures include unbiased coins and fair dice, while examples of unfair structures include biased coins and loaded dice. Anything that is the subject of probabilistic events can be regarded as a probabilistic structure, regardless of whether it is tangible or intangible.
B: "Actor." It makes A a dynamic state and actualizes its inherent probability. If the state is stationary and remains constant, such as with dice or coins, the inherent probability is not actualized unless B makes it a new dynamic state. Also, if A is always in a dynamic state, such as with the weather, B is not necessary, but it can be treated as a random and fair B. An actor has a frequency of actualization, and if it does not affect the inherent probability of A when it is actualized, it can be considered fair actor, otherwise it is an unfair actor. Examples of unfair actors include dealers who cheat with tricks using fair dice or cards in gambling.
C: "Observer." It observes the inherent probability of A actualized by B. C has an observation opportunity and an observed quantity for each opportunity. Since probability is a concept that deals with the credibility and frequency of "unknown objects," it only becomes an event through "observation." An observer has an observational ratio, and if it does not affect the results of A actualized by B during observation, it is fair, otherwise it is an unfair observer. An unfair observer has conditions or biases in its observation, such as when the observer closes its eyes and only opens them when the result is "heads" when a fair coin is tossed, making the observation ratio unequal.
…
P: The subjective probability of an observer when probabilistic structure is eventized by an actor and the observer observes it.
P = A x B x C
0 ≦ P ≦ 1.
Thinking about the problem:
As previously mentioned, the main issue in the Sleeping Beauty problem is the difference between the equal underlying probabilities of Heads and Tails for a fair coin and the observation ratio experienced by the observer (Sleeping Beauty), which is 1:2 for Heads and Tails.
The interpretation of this difference in observation ratios determines whether Sleeping Beauty assigns a subjective probability of 1/2 or 1/3 to the coin landing on heads.
The following diagram illustrates the observational ratio (chances) of "heads" and "tails" in a 1:2 relationship for both cases.
The perspective that assigns a subjective probability of 1/3 to the coin landing on heads from Sleeping Beauty's viewpoint interprets the difference in observation ratios as follows:
While the observation quantity for each instance of heads and tails is considered fair, the overall observation quantity is different (heads:tails = 1:2).
On the other hand, the perspective that assigns a subjective probability of 1/2 to the coin landing on heads interprets the overall observation quantity of heads and tails as fair, but the observation quantity per instance is considered to be different (heads:tails = 2:1).
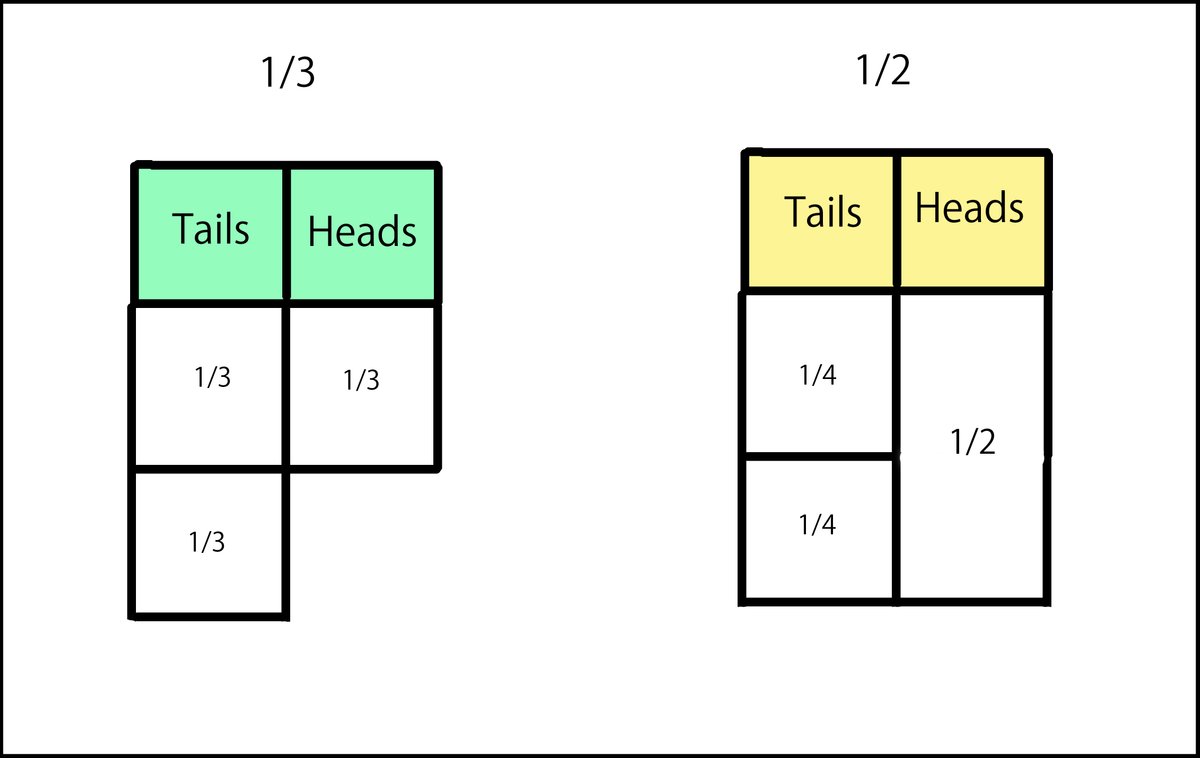
The perspective that assigns a subjective probability of 1/3 to "heads" in the Sleeping Beauty problem suggests that the observation quantities for each observation opportunities of "heads" and "tails" are equal, thereby making the event frequencies of a fair coin, determined by the actor, fair. This perspective considers the difference in observation ratios as a difference in overall observation quantities, indicating an unfairness in the observation process.
On the other hand, the perspective that assigns a subjective probability of 1/2 to "heads" in the Sleeping Beauty problem views the overall observation quantities of "heads" and "tails" for a fair coin as equal. However, it acknowledges that the observation quantities for each observation opportunities of "heads" and "tails" are different.
This perspective suggests that the event frequencies of "heads" and "tails" for a fair coin are different, implying an unfairness in the event frequencies determined by the actor.
In other words, the perspective that assigns a subjective probability of 1/3 to "heads" in the Sleeping Beauty problem signifies an unfairness on the part of the observer (Sleeping Beauty), while the perspective that assigns a probability of 1/2 implies an unfairness on the part of the actor (the experimenter).
…
Now, the question of which perspective is valid becomes a matter of discussion. However, in the explanation of the Sleeping Beauty problem, the fairness of the coin is explicitly stated, while the fairness of the experimenter (the actor) and Sleeping Beauty (the observer) is not specified. Therefore, within the framework of this thought experiment, it is possible to consider both perspectives, assigning a probability of 1/2 to reflect the potential unfairness of the actor and assigning a probability of 1/3 to reflect the potential unfairness of the observer.
However, the main purpose of this experiment is to explore the subjective probabilities and their changes in Sleeping Beauty's situation of conditional sleep and awakening. Arguing that the probability should be 1/2 based on the unfairness of the experimenter does not seem to align well with the main objective of the problem. Specifically, this means that the subjective probability of heads being 1/2 for Sleeping Beauty is a result of the experimenter manipulating the coin toss. Sleeping Beauty cannot deny it unless the fairness of the experimenter is guaranteed.
Therefore, it can be argued that the given conditions of this problem are insufficient to determine Sleeping Beauty's subjective probability. A reasonable modification would be to add the statement "the experimenter tosses the coin fairly" to the setup, in addition to the assumption of a "fair coin." By doing so, the difference in observation ratios can be attributed to the unfairness on the part of the observer, and the awakened Sleeping Beauty can determine a subjective probability of 1/3 for the coin landing on “heads.”
Alternatively, to maintain the setup of this problem, it is necessary to begin Sleeping Beauty's response to the experimenter with a question like the following:
…
Experimenter: What is the probability, from your perspective, that the coin I tossed while you were asleep landed on heads?
Sleeping Beauty: To answer that, I need to ask you a question.
Experimenter: What is it?
Sleeping Beauty: Did you toss the coin fairly? If so, then the probability of heads for me would be 1/3.
Experimenter: Why is that?
Sleeping Beauty: The intrinsic probability of heads for a fair coin, which remains constant, is always 1/2. It was also 1/2 for me before falling asleep. However, due to the conditions of the experiment involving sleep and awakening, the observation ratio of heads and tails upon waking became ‘heads : tails = 1:2.' However, there are two possible interpretations of this.
One interpretation is to assign a probability of 1/2 for (Monday: heads), 1/4 for (Monday: tails), and 1/4 for (Tuesday: tails). In this case, the subjective probability of heads for me would be 1/2. However, since the observation quantity of a single heads is equal to the combined observation quantity of two tails, the event frequency of the coin becomes unfair. This implies that your coin toss was unfair.
If you tossed the coin fairly, the observation quantities for heads and tails should be equal for each observation opportunity. If the coin is fair and you tossed it fairly, then the difference in observation ratios must be interpreted as a loss of fairness on my part as the observer. With an observation ratio of 1:2 for heads and tails, and equal probabilities for each observation opportunity, the probabilities for (Monday: heads), (Monday: tails), and (Tuesday: tails) would all be 1/3.
Therefore, if you tossed the coin fairly, the subjective probability of heads for me would be 1/3.
Summary of the problem:
What we can learn from this thought experiment is that we often handle the concept of probability quite vaguely and roughly.
Specifically, when considering the problem of a "fair coin," we tend to immediately assume that the probabilities of heads and tails are both 1/2. However, for a probabilistic event to occur, there must always be three elements involved: the coin itself (a probabilistic structure), the person flipping the coin (an actor), and the person observing the outcome of the coin toss (on observer). All three of these elements can be either fair or unfair, as demonstrated by this problem.
We are well aware from experience that the probabilistic structure and the actor can be unfair. For example, we know of cases such as a biased coin or a person cheating in a game. However, the unfairness of the observer is often not given much consideration. Especially when the observer is oneself, people tend to overlook questioning their own fairness.
Since individuals can only perceive the world from their own perspective, the unconditional belief of being a fair observer may be something that naturally occurs in everyone.The unconditional belief of being a fair observer oneself is the most crucial and intriguing aspect in dealing with subjective probabilities, as demonstrated in the "Sleeping Beauty problem."
What's even more fascinating is that this unconditional belief in one's own fairness can also be contemplated as a question of identity.
Probability ans identity:
Looking at it from Sleeping Beauty's perspective, this issue can also be viewed as a matter of her identity, as the fairness of her role as an observer is compromised by the "sleep and awakening" conditions in the experiment.
Despite the fact that the coin, which has an equal probability of "heads" and "tails," undergoes fair event frequencies, the subjective probability of "heads" for the awakened Sleeping Beauty changes to 1/3. Sleeping Beauty is compelled to interpret this as a loss of her fairness as an observer.
If we can state as a fact that the event frequencies of the fair coin are equal for "Heads" and "Tails" as 1:1, what perspective, position, or spacetime would be necessary to observe an observation ratio of 1:2 for the events?"
From Sleeping Beauty's standpoint, this transforms the question into an inquiry about her current self as an observer―what spacetime, position, she is in and whether she can be considered the same entity as her pre-sleep self.
Sleeping Beauty, who has lost her fairness as an observer, can be said to have also lost her identity as the observing entity for the coin used in the experiment, at the very least. Moreover, Sleeping Beauty can be said to occupy an irregular "spacetime" where she is capable of observing the subject that undergoes a 1:1 event ratio with a 1:2 ratio.
When considering the Sleeping Beauty problem in terms of the issue of identity, the relevance of "position and spacetime" arises because this thought experiment is composed of two independent probabilities. One pertains to the binary choice of heads or tails in the coin toss, while the other pertains to the binary choice of Monday or Tuesday for the day determination.
The events of the coin toss (Heads and Tails) represent a 1-dimensional spatial choice, while the determination of the day represents a 1-dimensional temporal choice.
Therefore, the possible states given to the awakened Sleeping Beauty correspond to the combinations of these two dimensions, namely (Heads, Monday), (Tails, Monday), and (Tails, Tuesday), forming a fundamental event combining spatial (1-dimensional) and temporal (1-dimensional) aspects.
As a result, Sleeping Beauty gains a perspective in a 3-dimensional space that encompasses and overlooks the combination events of the spatial (1-dimensional) and temporal (1-dimensional) dimensions.
Consequently, Sleeping Beauty acquires a viewpoint situated in a three-dimensional space that encompasses the combination of spatial (one-dimensional) and temporal (one-dimensional) events, allowing for comprehensive observation.
From Sleeping Beauty's perspective, the impression and sensation of this problem revolve around the questions of where she is and whether she remains the same entity as before falling asleep. The question posed to Sleeping Beauty is, "What is the probability of the coin landing heads, from your perspective?" However, the essence of this question can be seen as inquiring about the preservation of Sleeping Beauty's identity. If Sleeping Beauty maintains her identity as an observer, then the probability of the coin landing heads remains 1/2; otherwise, it becomes 1/3.
The Sleeping Beauty problem can be viewed from two different angles: as a probability problem and as an identity problem.
Probability and identity both involve dealing with certainty and degrees, and the Sleeping Beauty problem is particularly interesting because it highlights the intimate relationship between these two aspects.
23.05.19th
