
Road to Modularity - Deformation Theory of Galois Representations
This is just to record my progress, albeit being slow, in what I aim in the following article:
It really took time, but I had to ensure I cover various themes of Arithmetic of Elliptic Curves and some other topics, so I started reading "An Introduction to the Deformation Theory of Galois Representations" by Barry Mazur in "Modular Forms and Fermat's Last Theorem" on 17th November 2024.
In addition, I was unable to study at all for several days due to a high fever from the flu, but managed to read this document before the end of the year.
During my study, I occasionally read "Deformation of Galois Representations" by Fernando Q. Gouvêa in "Arithmetic Algebraic Geometry" (Brian Conrad, Karl Rubin Editors). This article is quite lengthy, but easier to read.
The following somewhat classical theorem is one of the foundations of Deformation Theory of Galois Representations:
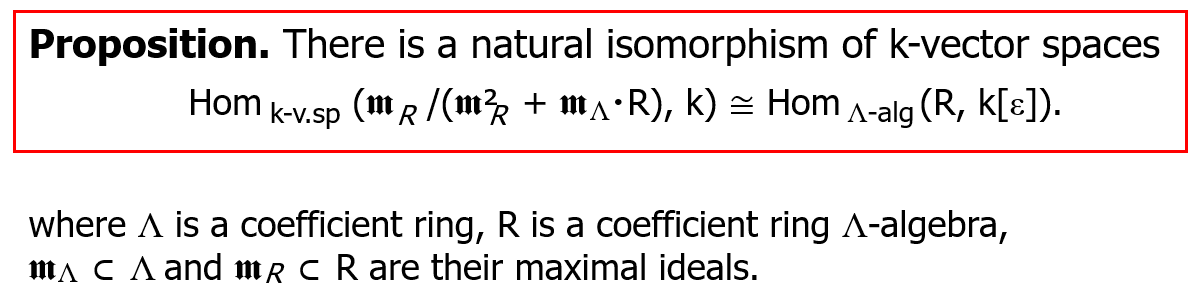
The Zariski tangent space is a basic construction that defines a tangent space at a point P on an algebraic variety V in Algebraic Geometry.
As Barry Mazur writes:
The key idea is to invoke the Λ-algebra k[ε] by the relation ε² = 0.
The algebra k[ε] is a k-vector space of dimension 2.
k[ε] = k ⨁ ε・k
The above k[ε] = k[t]/(t²) appears in classical deformation theory in Algebraic Geometry, an example of which known as "Deformation Theory of Complex Manifolds" by Kunihiko Kodaira is very famous.
Needless to say, this theory by Barry Mazur, being armed with category theory, is much more than the Zariski tangent space and this article talks a lot about several versions of representability of the deformation functor and deformation conditions.
The next article to study is "Explicit Construction of Deformation Rings" (by Bart de Smit and Hendrik W. Lenstra, Jr.), a relatively short one, then "Serre's Conjecture" (by Bas Edixhoven (RIP🙏))…
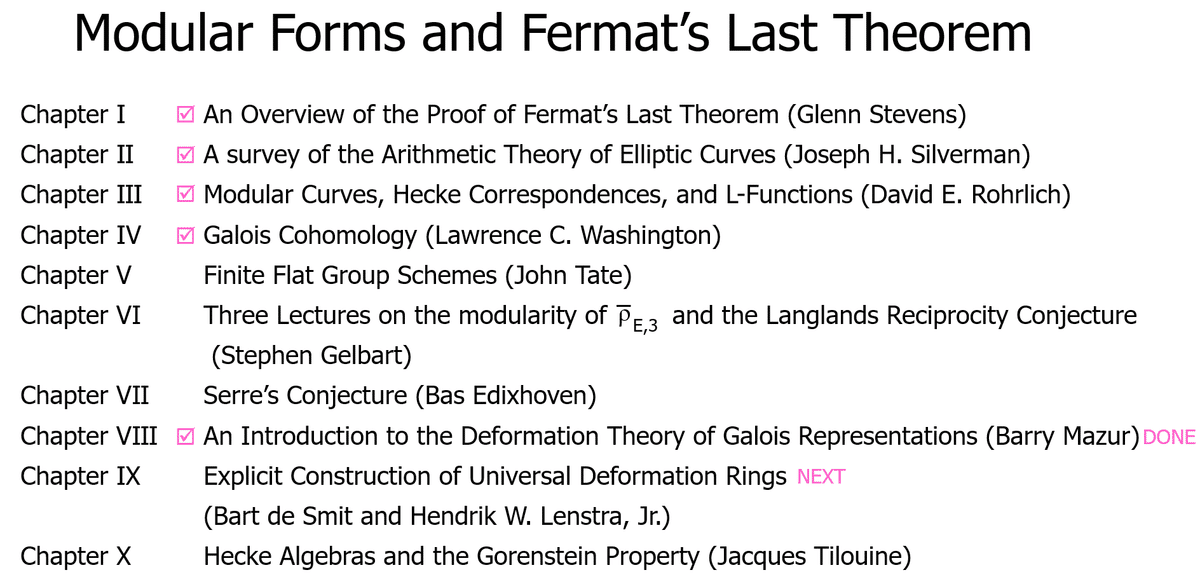

The road is (very) long, and it was to be expected... It CANNOT be easy❗

■
