Ready to tackle FLT (Fermat's Last Theorem) ?
Image Credit: The header image has been taken from Quanta Magazine (New Proof Distinguishes Mysterious and Powerful 'Modular Forms')
At the end of February this year (2024), I finished (re-)learning Algebraic Geometry using "Algebraic Geometry and Arithmetic Curves" by Qing Liu.
Before that, I had learned Class Field Theory and Elliptic Curves, and I just finished reading "A First Course in Modular Forms" by Fred Diamond and Jerry Shurman, though I admit that I did not meticulously look into every reference to understand claims without proof.

While "Modular Functions" interested me when I was a student, I found the famous textbook by prominent mathematician Gorō Shimura titled "Introduction to the Arithmetic Theory of Automorphic Functions" difficult to follow.

On the other hand, this book by Fred Diamond and Jerry Shurman is highly enlightening for me, though it was not easy.
There's an intriguing book review of this textbook by Fred Diamond and Jerry Shurman:
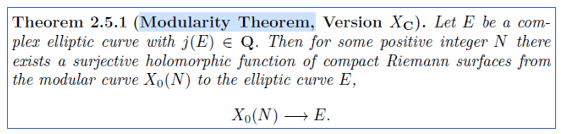
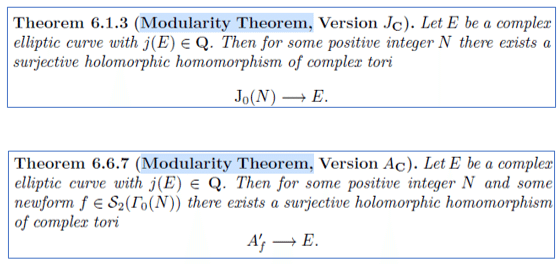
The title of the book is a bit deceptive, what it is really about is what used to be called the Taniyama-Shimura-Weil (or some subset of those names) conjecture, but now is often known as the Modularity Theorem. Most of this theorem was proved by Andrew Wiles (with help from Richard Taylor), who famously used his result to prove Fermat’s last theorem. More recently, the proof of the full theorem was completed by Fred, together with collaborators Christophe Breuil, Brian Conrad and Richard Taylor.
...
The book takes an interesting approach to the Modularity Theorem, not trying to actually prove it. The proof involves highly sophisticated mathematical technology, and really understanding it is still the province of experts. If one wants to try and learn this technology, two places to look are the volumes Modular Forms and Fermat’s Last Theorem and Arithmetic Algebraic Geometry, which are the proceedings of two different instructional conferences. Instead of trying to give a proof, Diamond and Shurman’s book explains exactly what the various related versions of the Modularity Theorem say. This covers a range of beautiful mathematical ideas, much of which hasn’t before had a particularly readable exposition. Until now, the main reference for some of this material has been Shimura’s Introduction to Arithmetic Theory of Automorphic Functions, a famously difficult text.
…
But this really is a wonderful book, making accessible parts of the really beautiful mathematics which mathematicians have been making great progress in understanding over the last decade.
The above book review says that Shimura's text "famously difficult", which means that my impression 45+ years ago had not been absurdly incorrect😎.
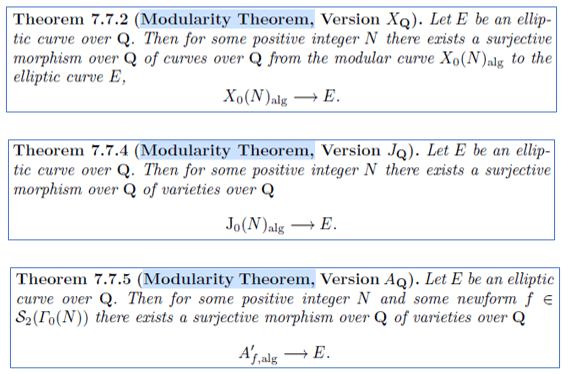
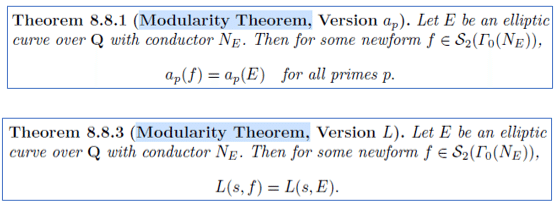
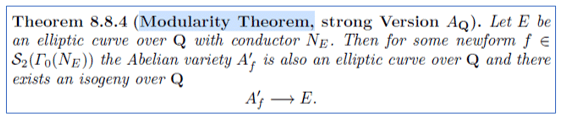
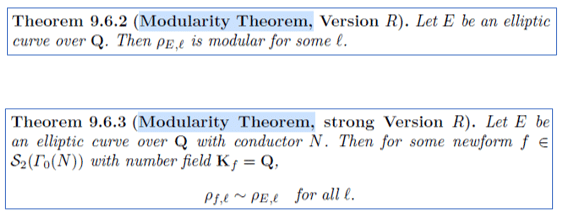
As mentioned also that "this book does not prove Modularity Theorem", it refers to several versions of "Modularity Theorem" without proof, but explains relationship among them.
Here are books mentioned in the above book review:


As I wrote in the aforementioned article "Jugendtraum…", I specialized in Algebraic Topology (#代数的位相幾何学), a branch of mathematics #数学 , however, I decided to study Number Theory (#数論) after retirement in order to understand the proof of FLT (#フェルマーの最終定理) by Andrew Wiles. I'm going to tackle with the books mentioned in the above book review, even though it will be highly challenging for me to follow every detail such as Euler System by Kolyvagin.
Last but not least, let me add a remark that the following book by Yves Hellegouarch is also enlightening especially with descriptions of history of problem solving.

In the proof of Fermat's Last Theorem, the following elliptic curve plays the key role:

This curve is called Frey curve, but also called Frey–Hellegouarch curve, because:
Yves Hellegouarch a introduit en 1969, en partant d'une hypothétique solution à l’équation de Fermat, la courbe elliptique E(a, b) :…
Its Chapter 5 Modular Forms is quite interesting albeit daunting style in 5.2 THE THETA FUNCTIONS… The chapter starts with "The Tyger", a poem by the English poet William Blake, published in 1794 as part of his Songs of Experience collection.
Then Section 5.5 is titled "THE FIFTH OPERATION OF ARITHMETIC", which comes from what Martin Eichler, a German mathematician, supposedly said : "There are five fundamental operations in mathematics. Addition, subtraction, multiplication, division and modular forms.”
It's not that important whether or not Martin Eichler actually made such a statement, but here are two references:
① Reference to the statement is found in an interview below.
ANDREW WILES: There's a saying attributed to Eichler that there are five fundamental operations of arithmetic: addition, subtraction, multiplication, division, and modular forms.
② On the other hand, "Modular Forms" (Bas Edixhoven, Gerard van der Geer and Ben Moonen) calls this statement apocryphal: