
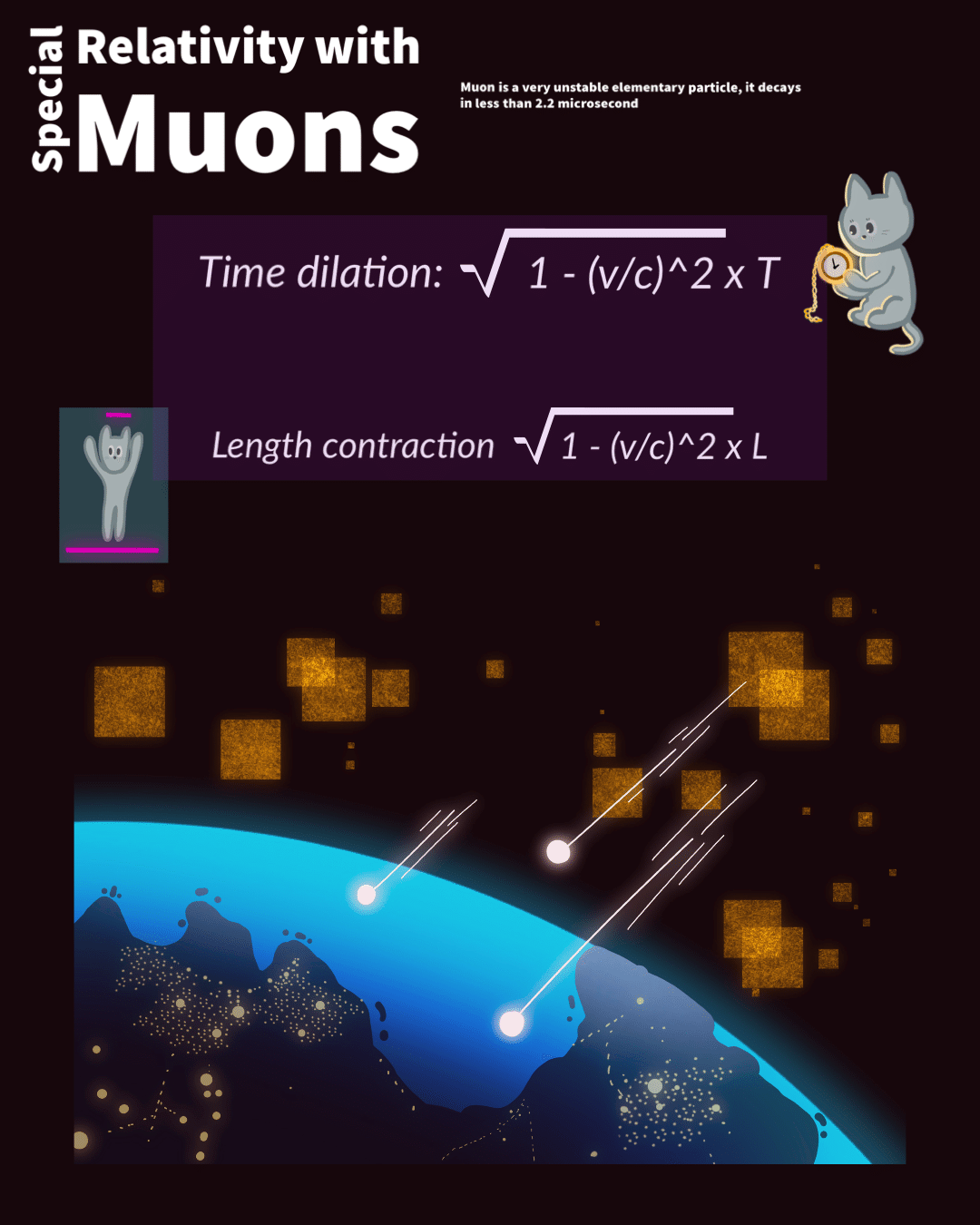
Special Relativity with Muons 1
I have a feeling that, when you hear science theories that some very famous scientists have discovered, most of you can’t help but think it’s too difficult to understand.
Especially, when it is Einstein.
The theory that I am going to talk about here in this article is made by Einstein and does have some extraordinary ideas that may blow your minds. But mathematically, it’s not too challenging so I believe most people can dive into it. (Unless you won’t dive in too far;)
I believe it is one of many theories of physics that exceptionally feel like science fiction. I mean, it’s astonishing.
(英語のブログです。ヨビノリさんの動画を参考に書いています。The link below will bring you to a youtube video where I learnt this theory. It is in Japanese tho.
[相対性理論とは何か]を理解する易しい授業)
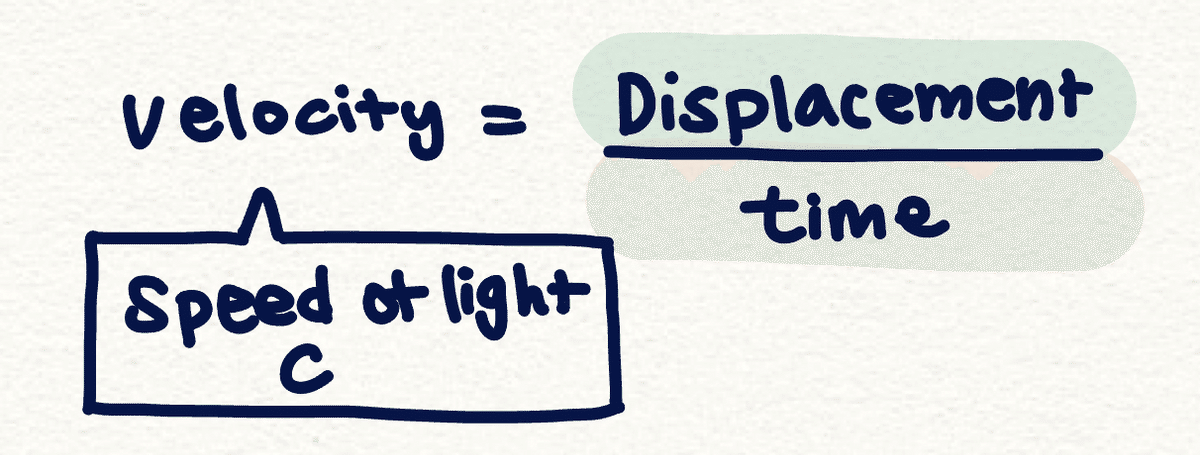
V=S/T
Velocity = Displacement/time
I am pretty sure that most of you know this equation. Velocity means how far an object can travel in a given period of time. (How fast an object is moving through space in a certain direction.)
We can also apply this equation to some extreme conditions, such as when the velocity is the speed of light “C” (the ultimate speed limit of the universe), which is 3 00,000km/s.
As C is always constant* it will never be changed. Instead, we can work out from this equation that it will be either Space (displacement) or Time that will be changed.
*There is an important rule of the universe that we need to remember here:
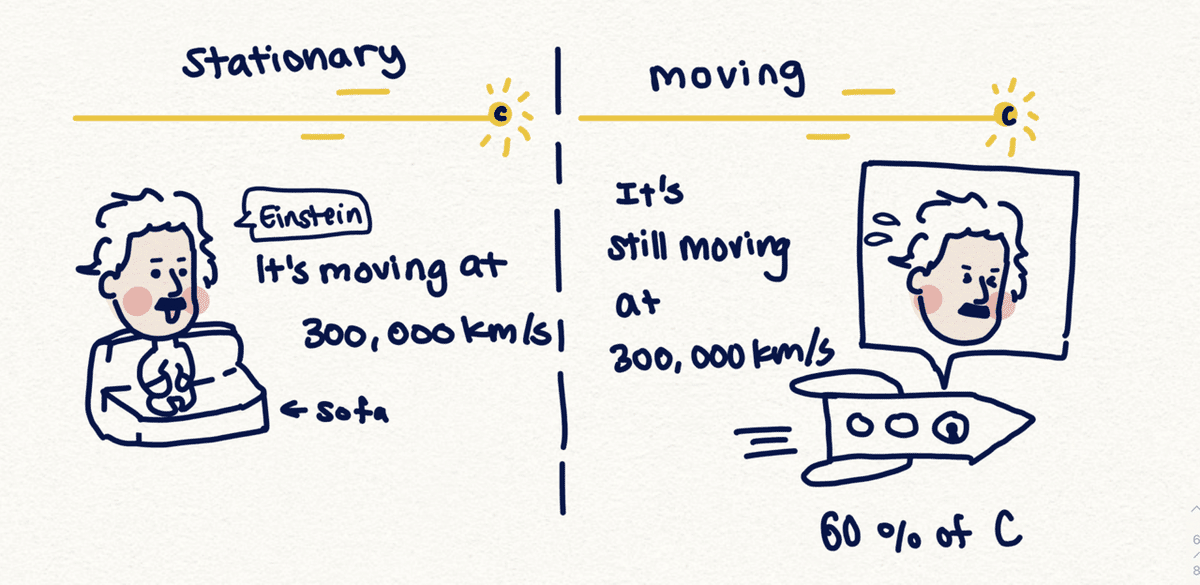
The speed of light C is constant, independent of the speed of the observer.
What does this mean?
It means C will always be 300,000km/s relative to all the observers. The measurement of C you make while you are sitting on your sofa is exactly equal to the measurement of C you make while you are moving close to the speed of light in the spaceship.
This is a counterintuitive idea and difficult to get our head around. It is because this does not happen in the normal life we experience, where V is nowhere near C. Let’s say you are riding on a bike at 5m/s and watching a train passing you by at 10m/s. Because you, the observer, are moving with the train, the train will seem to be moving at 5m/s away from you.
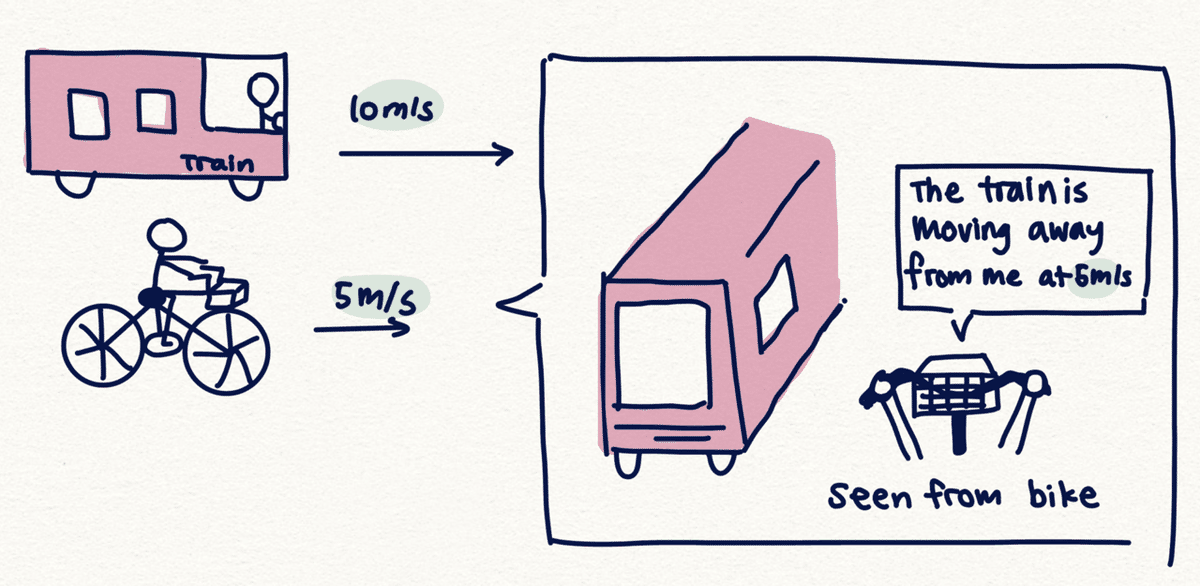
But this subtraction of velocity does not work when C comes in. If you are observing a light beam, no matter how slow or fast you are moving relative to it, C will always be constant.
Back to V=S/T
Previously, I said that because C is unchanged, it is either space or time that changes.
Let’s dive in to how “time” changes:
— Time dilation —
To understand this concept, let’s think of a little experiment where we observe a light beam travelling inside a train vertically. (The train is moving at the constant speed)
I am inside the train, while you are standing outside.
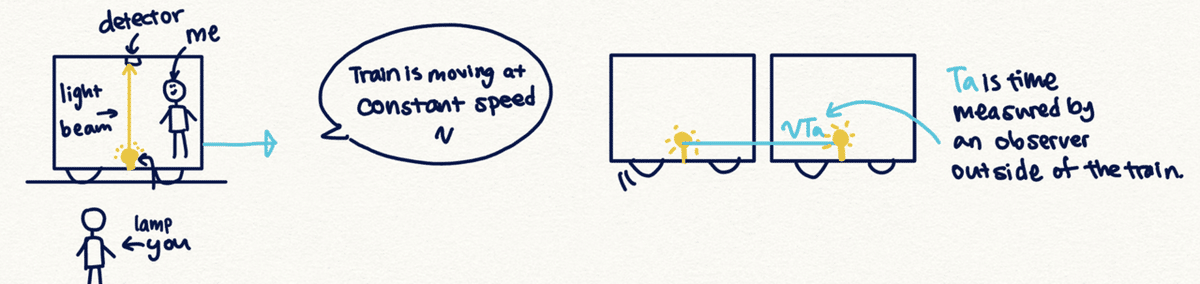
We want to know how “Time” changes, so we individually use a stopwatch to measure time taken for a light beam to travel from the lamp to a detector on the ceiling of the train. Let’s say that while we measure the time, the train moves horizontally in a constant speed V for T(a) seconds.
This means that during the experiment the train moves V x T(a) meters.
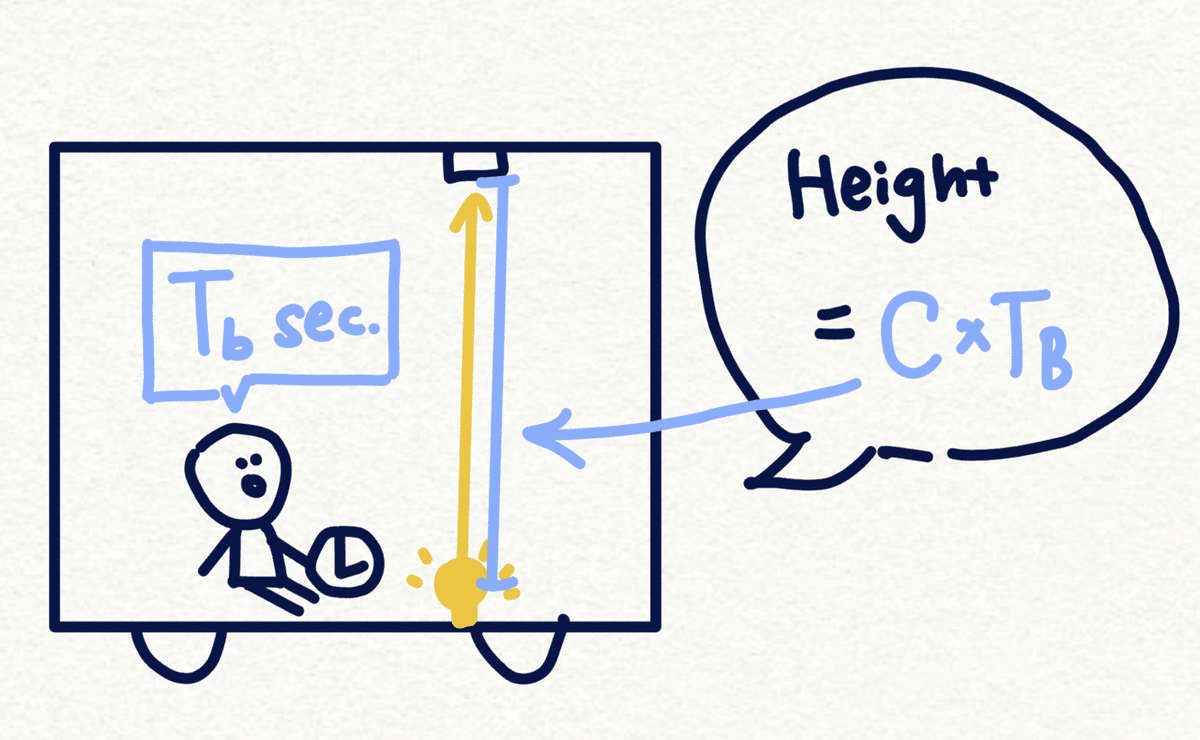
As I am standing inside the train to observe the light, I see the light beam travel to the detecter vertically.
Let’s say my stopwatch says it took T(b) seconds for the light to travel from the lamp to the detector.
So, the displacement (or the height of this train) is
S(vertical) = C x T(b)
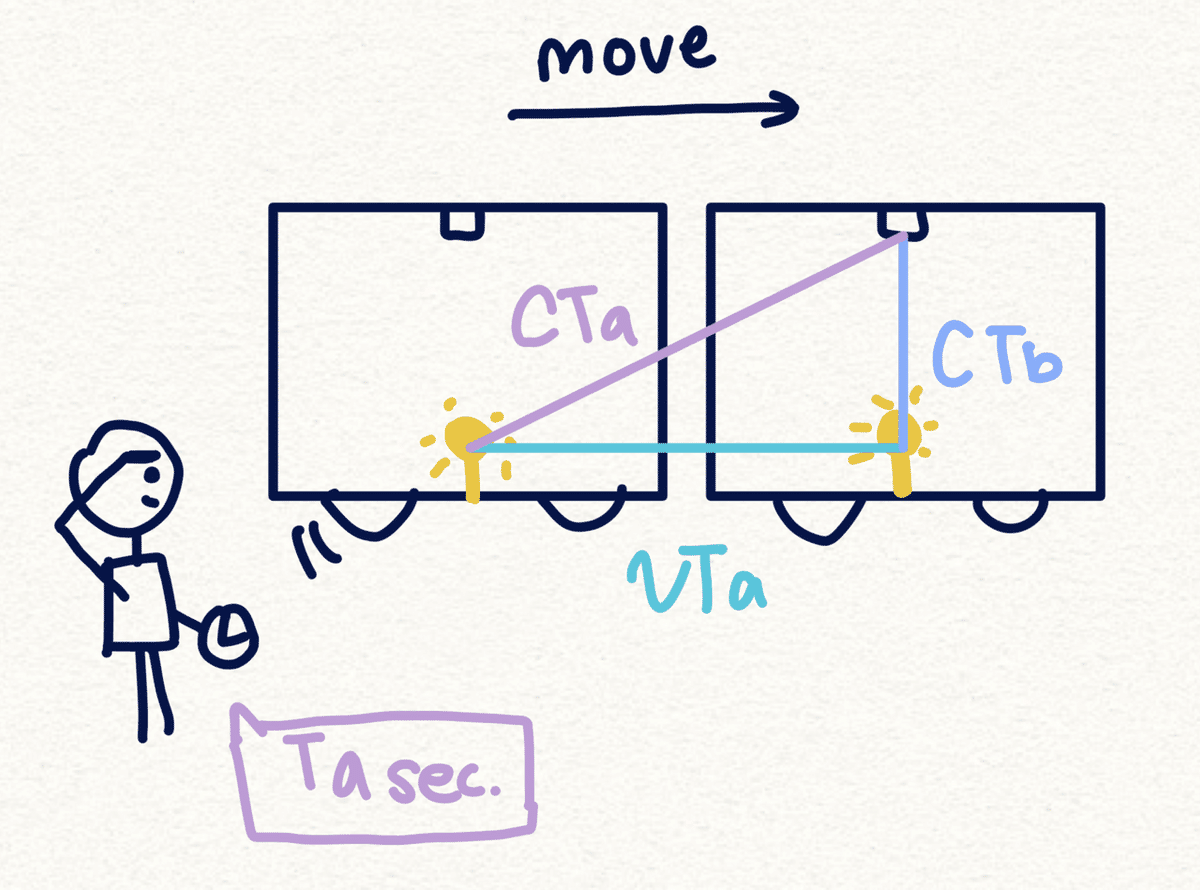
From your perspective as a person standing outside, it will be a little different because the train itself is moving while the light beam travels to the ceiling.
Therefore you see the light beam travelling diagonally from the lamp to the detector.
Let’s say that you measure the time T(a) on your stopwatch.
Just like how we calculated the distance before, we can work out that
S(diagonal) = C x T(a)
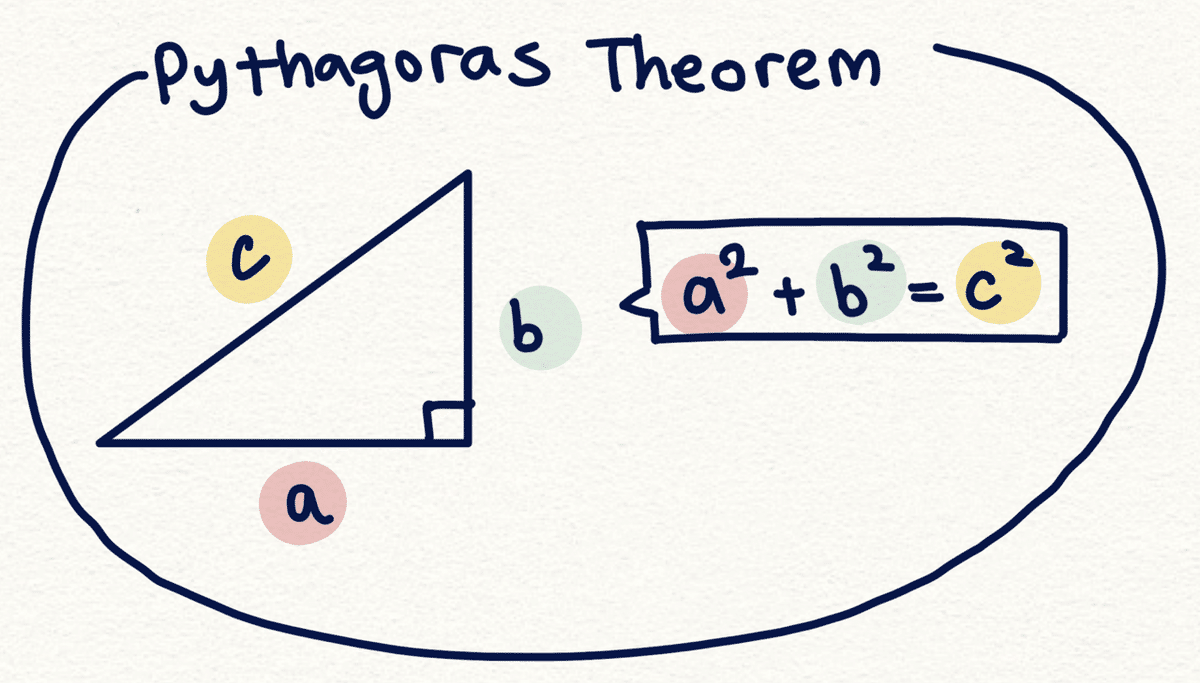
Now, to understand these equations, I introduce to you the well known Pythagoras theory, where the square of horizontal length a multiplied by the square of vertical length b is equal to the square of hypoteneous.
Using this theorem with our results, we get
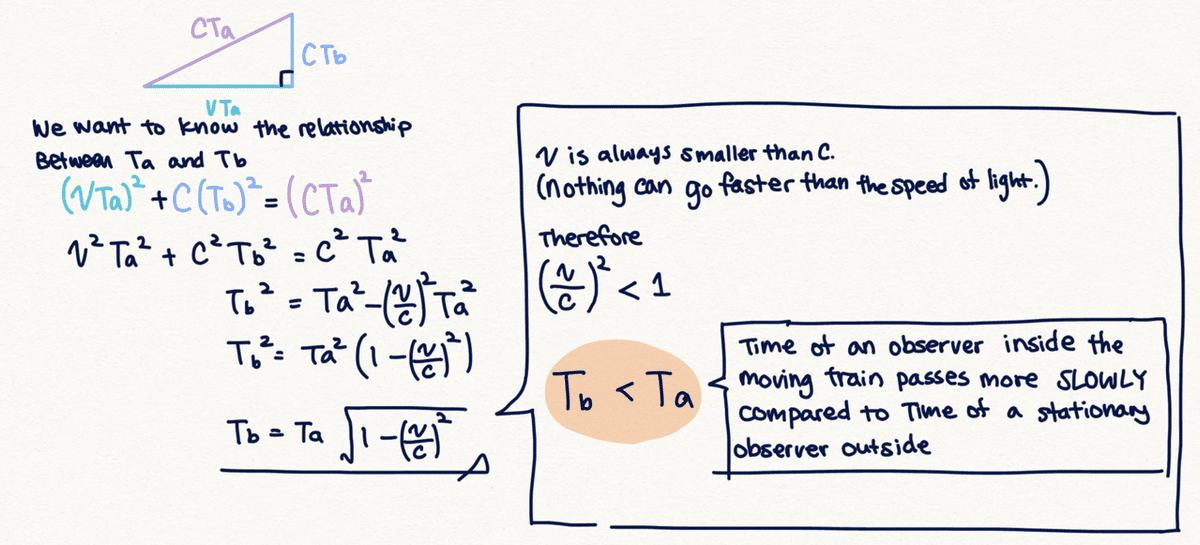
In this equation we have the time that you have measured on the left hand side and the time that I have measured on the right hand side. If we think that time runs equally everywhere, we should get T(a)=T(b) but it clearly isn’t.
T(b) seems to be multiplied by something.. but what is it?
In a nutshell, square root of (1-(V/C)^2) is always smaller than 1 which means the time passing on your stopwatch is always faster than the time passing on my stopwatch.
When I am moving through space with the train, time passes more slowly compared to how fast time passes in your perspective as a stationary observer.
Time that you experience is dilated relative to mine.
Let’s think of a more specific example, where the train that I am riding on is moving at 80% the speed of light. When we do some maths, we get a result that my time is passing at 60% the rate of your time. When your stopwatch says it’s been an hour since the experiment has started, my stopwatch will say it has only been 36 minutes.

It’s natural to think that time passes equally everywhere, but it is not absolute at all. It flows differently when things get extreme and move close to the speed of light.
The famous theoretical physicist, Albert Einstein has said,
Distinction between past, present and future is only a stubborn, persistent illusion.
In this blog I have just talked about how “time” differs when relative
speed is different. However, there is another half of this story. Remember the first thing that I said? S = V/T. Yes. There is another whole story of space (displacement) waiting to unfold.
おまけ
I now have a total of 5 paintings of the images that JWST has taken! I am excited to see a lot more images coming up soon!

Anticlockwise order from the bottom right
1) Carina Nebula
2) Southern Ring Nebula
3) Stephan’s Quintet
4) SMACS 0723
5) Cartwheel Galaxy
