書記が数学やるだけ#732 基本対称式とニュートンの恒等式

基本対称式の復習を兼ねて，ニュートンの恒等式について見ていく。

問題

説明

ニュートンの恒等式（ジラール-ニュートンの公式）からは，任意の対称多項式がべき和で表現できることがわかる。このことはガロア理論をはじめとしてさまざまな分野に応用可能である。

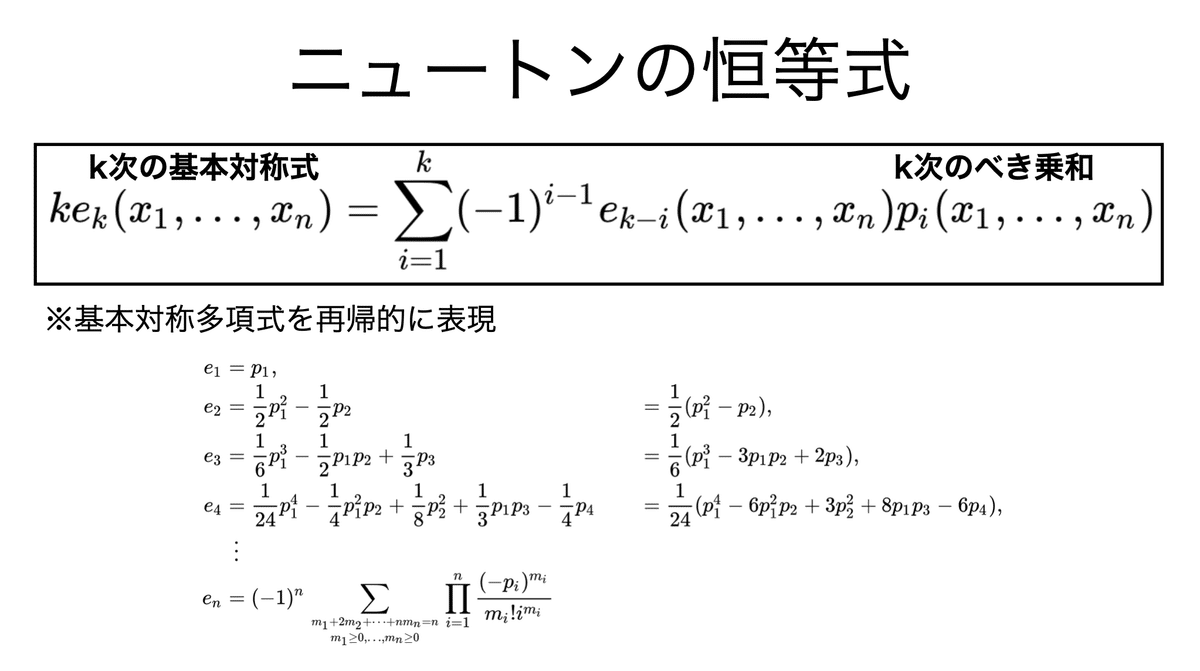
解答

東大の簡単な問題から，基本対称式を用いた漸化式の解法を復習。

基本対称式と差積の関係について一例を示す。

ニュートンの恒等式の証明を行う。ここで鍵となるのはべき級数の公式である。

本記事のもくじはこちら：