
【理系・海外大学院】数学・物理を日本語⇄英語でざっくりまとめてみた
先月出願した大学院の書類選考に通り、2次選考の数学・物理・地球物理の口頭試験の案内が来ました。Zoomで30分、教授2人対私…。今日からちょうど1週間後(°_°)
追記:面接後、その場で合格いただきました💮
noteでノート作るの大好きマンなので、テンション上げるために(?)1、2回生の総復習(広く浅め)をここにまとめます。noteだと移動中にスマホでパパッと見直せるのも良い⭕️
理系で海外の大学院行きたいけど、「数学も物理も英語でやったことない!」って人のお役に立てればと思います。留学予定がないとしても、英語で論文読むのに役立つかも!
ただ、すごく基礎的な内容なので、①英語で数学・物理の話を適切な用語を使って説明できるようになること、②忘れかけていた数学・物理の基礎知識を思い出すのに辞書的に眺めてもらうのに最適です。
英語で「固有値」「角運動量」ってパッと言えるか?で詰まった人にはおすすめかも?
(答え:eigenvalues, angular momentum…案外知らん。)
【数学】そもそも数式読めない説😬
まず、誰もが暗唱したであろう2次方程式の解の公式を英語で声に出して読んでみてください!
日本語:xイコール2a分のマイナスbプラスマイナスルートb2乗マイナス4ac
英語:Quadratic formula
x equals negative b, plus or minus the square root of b squared minus four ac, all over two a
1番の違いは、分数を
・日本語:(分母)分の(分子)👆
・英語:(分子: denominator)over(分母: numerator)👇
と、上下反対の順番で読むこと!!
squared:2乗、とか個々の単語は出てくる度Excelに表でまとめた!
【数学】線形代数(Linear algebra)
全ての用語を解説するとキリがないので、項目に関連する数学用語を「日本語(English)」で列挙しています!
ベクトルと行列(Vectors and Matrices)
・Vector: a quantity with magnitude and direction
ex) a vector in n-dimensional space
・Matrix: two-dimensional array of numbers arranged in rows and columns, used to represent linear transformations
ex) 2 times 3 matrix
・ベクトル: 大きさと方向を持つ量(例)n次元ベクトル
・行列: 数値を行と列に並べた二次元配列で、線形変換を表現するために用いられる。(例)2×3行列
加減算(addition and subtraction)、スカラー倍(scalar multiplication)、行列積(matrix multiplication)
固有値と固有ベクトル(Eigenvalues and Eigenvectors)
・Eigenvalue: a scalar that characterizes a linear transformation by scaling a vector without changing its direction. It act as scaling factors for their corresponding eigenvectors.
・固有値: ある線形変換を行った際に、ベクトルの方向を変えずに、その大きさだけを拡大または縮小するスカラー値。対応する固有ベクトルのスケール係数。
特性方程式(Characteristic equation)、行列式(Determinant)
線形変換(Linear Transformations)
・Linear transformation: a mapping that relates elements of one vector space to another, satisfying additivity and homomgeneity.
・線形変換: ベクトル空間の要素を他のベクトル空間に対応付ける写像であり、加法性f(x+y)=f(x)+f(y)と斉次性f(ax)=a f(x)を満たす。
回転変換(Rotation Transformation)、スケーリング変換(Scaling Transformation)、剪断変換(Shear Transformation)
正方行列(Square matrix)、単位行列(Identity matrix)、対角成分(Diagonal elements)、逆行列(Inverse matrix)、転移行列(Transpose matrix)、入れ替える(swap)、直行行列(Orthogonal matrix)、対称行列(Symmetric matrix)、対角行列 (Diagonal Matrix)、ヘルミート行列 (Hermitian Matrix)、射影行列 (Projection Matrix)
・行列式が0の場合、逆行列は存在しない。(If determinant det(A) = 0, the inverse matrix does not exist.)
・転置行列が逆行列と一致する場合、直交行列。(If the transpose matrix is equal to its inverse, that is orthogonal matrix.)
・ヘルミート行列は、複素数成分を持つ行列で、転置共役が自分自身と等しいもの。(A Hermitian matrix is a complex-valued matrix that is equal to its conjugate transpose.)
…この辺は数式を見た方が早いので、上記の単語を使って自分で定義を説明する練習をしてみてください。
【数学】微積分(Calculus)
微分(Differentiation)
The derivative of a function f(x) is defined as the limit of the ratio of the change in the function Δy to the change in the variable Δx as Δx approaches 0
関数 f(x) の微分係数は、変数 x の変化 Δx に対する関数の変化 Δy の極限として定義される。
和の法則(Sum rule)、積の法則(Product rule)、商の法則(Quotient rule)、連鎖律(Chain rule)
偏微分(Partial derivatives)、常微分方程式(Ordinary differential equations, ODEs)、偏微分方程式(Partial differential equations, PDEs)
積分(Integration)
Integration calculates the cumulative value of a function f(x) and is divided into indefinite and definite integrals
積分は、関数 f(x) の累積的な値を計算するもので、不定積分と定積分に分けられる。
和の法則(Sum rule)、スカラー倍の法則(Scalar Multiplication rule)、部分積分(Integration by parts)、置換積分(Substitution rule)
・微分と積分は互いに逆操作である。(Differentiation and integration are inverse operations.)
グリーンの定理(Green's theorem)
For a closed curve C and its enclosed region S in a plane, Green's theorem relates a line integral (circulation) to a double integral (curl)
平面上の閉曲線 C とその内部領域 S に対し、線積分(循環)と面積分(回転)を関連付ける定理
ナブラ演算子∇(Nabla, Del)
1. Gradient: applied to a scalar field f(x,y,z) to produce a gradient vector
2. Divergence: applied to a vector field F(x,y,z) to produce a scalar field
3. Curl: applied to a vector field F(x,y,z) to produce another vector field
勾配: スカラー場に適用し、勾配ベクトルを得る
発散: ベクトル場に適用し、スカラー場を得る
回転: ベクトル場に適用し、別のベクトル馬を得る
発散定理(Divergence theorem)
For a volume V and its boundary surface S, the divergence theorem relates the volume integral of the divergence of a vector field ∇・F to the net flux F・n across the surface
体積 V とその境界となる閉曲面 S に対して、ベクトル場の発散 ∇・F の体積積分と閉曲面上のフラックス F・n の面積分を関連付ける定理
流体力学(Fluid dynamics)、ガウスの法則(Gauss's law)
ストークスの定理(Stokes' theorem)
For a surface S and its boundary curve C, Stokes' theorem relates the surface integral of the curl (vorticity) of a vector field F to the line integral (circulation) around the curve
曲面 S とその境界となる閉曲線 C に対し、ベクトル場 F の回転(渦度)の面積分と線積分(循環)を関連付ける定理
フーリエ解析(Fourier Analysis)
Fourier analysis decomposes any periodic function or signal into a sum of sines and cosines.
The Fourier transform converts a function from the time domain to the frequency domain.
フーリエ解析は、任意の周期関数や信号を正弦波と余弦波の和に分解して表現する手法。
フーリエ変換では、時間領域の関数を周波数領域に変換する。
離散フーリエ変換(Discrete Fourier transform, DFT)、高速フーリエ変換(Fast Fourier transform, FFT)、畳み込みの定理(Convolution theorem)
ラプラス変換(Laplace transform)
The Laplace transform converts a function f(t) into a complex-plane function F(s).
ラプラス変換は、関数 f(t) を複素平面上の関数 F(s) に変換する手法。
・微分方程式を解くには、まず方程式全体をラプラス変換し、代数的な式に変形する。そして、解を複素平面上でF(s)の形で求め、逆ラプラス変換をし、元の時間領域の解を求める。
(To solve differential equations, first take the Laplace transform of the entire equation and simplify into an algebraic expression. Then, solve for F(s) in the complex plane and apply the inverse Laplace transform to find the original solution f(t) in the time domain.)
例文作ってみたけど、あってるかな?
(数学的にも英語的にも😂)
【物理】古典力学(Classical Mechanics)
ニュートンの運動法則(Newton's Laws of Motion)
1. Law of Inertia: An object remains at rest or in uniform motion unless acted upon by a force.
2. Law of Motion: Force equals mass times acceleration (F = ma).
3. Law of Action and Reaction: For every action, there is an equal and opposite reaction.
慣性の法則: 静止または等速直線運動を保つ。
運動の法則: 力は質量と加速度に比例 (F = ma)。
作用・反作用の法則: ある物体が他の物体に力を加えると、同じ大きさで逆向きの力を受ける。
運動量保存則 (Conservation of Momentum)
The total momentum of a closed system remains constant unless acted upon by an external force.
閉じた系では、全運動量は外力が作用しない限り一定に保たれる。
エネルギー保存則 (Conservation of Energy)
Energy can change forms (e.g., kinetic to potential energy) but remains constant in a closed system.
エネルギーは形を変えることはあっても、全体として一定に保たれる(運動エネルギー、位置エネルギーなど)。
運動方程式 (Equations of Motion)
Differential equations describing position, velocity, and acceleration.
・Linear motion
・Rotational mortion
物体の位置、速度、加速度を記述する微分方程式。
・直線運動
・回転運動
↑この辺の単語は、何を研究をするにしても出てくるはず!たぶん。
【物理】熱力学(Thermodynamics)
熱力学の基本法則 (Laws of Thermodynamics)
1. First Law:
The law of energy conservation. The heat added to a system(Q), equals the change in internal energy(U), plus the work done by the system(W)
Q=ΔU+W
エネルギー保存の法則。系に加えられる熱Qは、内部エネルギーUの変化と仕事Wの和に等しい
2. Second Law:
Natural processes increase the entropy(S) of a system. A perfectly efficient heat engine is impossible.
自然界のプロセスはエントロピー S(乱雑さの尺度)を増加させる方向に進む。完全効率の熱機関は存在しない。
(エントロピーS:系の乱雑さ。仕事に使えないエネルギー。
エンタルピーH:系の総熱量。UとPVの合計。)
3. Third Law:
As the temperature approaches absolute zero, the entropy of a system approaches a constant value.
温度が絶対零度に近づくと、系のエントロピーは一定の値に近づく。
0. Zeroth Law:
If two systems are in thermal equilibrium with a third system, they are in thermal equilibrium with each other.
2つの系が第三の系と熱平衡にあるなら、それらは互いに熱平衡にある。
熱力学ポテンシャル (Thermodynamic Potentials)
1. 内部エネルギー (U / Internal Energy)
The total energy contained within a system, including kinetic and potential energies of particles.
dU=TdS-PdV
系の中に含まれる全エネルギー(粒子の運動エネルギーや位置エネルギーを含む)。
2. エンタルピー (H / Enthalpy)
Represents the total heat content of a system at constant pressure.
Commonly used to describe heat transfer in chemical reactions and phase transitions under constant pressure.
H=U+PV
一定圧力下での系の熱エネルギーの総量。化学反応や相転移の熱移動を記述する際に用いられる。
3. ギブズ自由エネルギー (G / Gibbs Free Energy)
The maximum work that a system can perform at constant temperature and pressure.
Determines whether a process or reaction will occur spontaneously.
G=H-TS
ΔG=0: Chemical Equilibrium
一定温度・一定圧力下で、系が行うことができる最大の仕事。プロセスや化学反応が自発的に進行するかどうかを判定する。ΔG=0:化学平衡。
4. ヘルムホルツ自由エネルギー (F / Helmholtz Free Energy)
The amount of energy available to do work at constant temperature.
Used in systems involving thermal and mechanical interactions.
F=U-TS
一定温度条件下で利用可能なエネルギー。熱的および機械的相互作用を含む系で使用される。
その他
等温過程 (Isothermal Process): 温度一定(T=const.)。
断熱過程 (Adiabatic Process): 熱の出入りなし(Q=0)。
等圧過程 (Isobaric Process): 圧力一定(P=const.)。
等体積過程 (Isochoric Process): 体積一定 (V=const.)。
相転移 (Phase Transitions): 固体、液体、気体 (solid, liquid, and gas)。
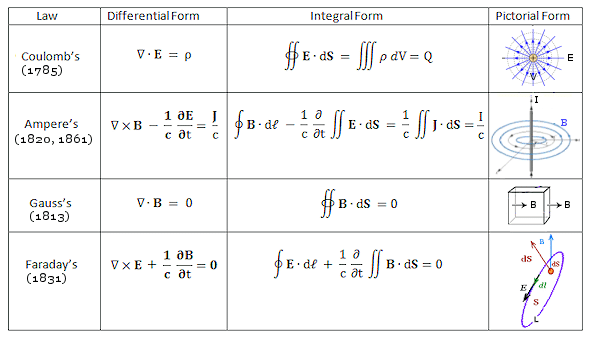
【物理】電磁気学(Electromagnetism)
言葉で説明するには、積分形の概念を示した方がわかりやすいと思います。が、∇の意味を理解するために微分形(🐷びぶん)も並べました。英語で概念を説明するのが目的なので、式は省略しました。
クーロンの法則 (Coulomb's Law)
The electrostatic force between two point charges is proportional to the product of the charges and inversely proportional to the square of the distance between them.
2つの点電荷間に働く静電気力は、それぞれの電荷の積に比例し、距離の二乗に反比例する。
次にマクスウェル方程式(Maxwell's Equations)👇
1. ガウスの法則(電場) (Gauss's Law for Electric Fields)
The electric flux through a closed surface is proportional to the total charge enclosed within the surface.
🐷The divergence of the electric field is proportional to the charge density.
閉曲面を通過する電場のフラックスは、閉曲面内の総電荷に比例する。
🐷電場の発散は電荷密度に比例する。
2. アンペール・マクスウェルの法則 (Ampere-Maxwell Law)
The circulation of the magnetic field around a closed loop equals the sum of the current and the time rate of change of the electric flux.
🐷The curl of the magnetic field is proportional to the current density and the time rate of change of the electric field.
磁場の循環は、電流と時間的に変化する電場の和に比例する。
🐷磁場の回転は電流密度と時間的に変化する電場の和に比例する。
3. ガウスの法則(磁場) (Gauss's Law for Magnetic Fields)
The magnetic flux through a closed surface is always zero (there are no magnetic monopoles).
🐷The divergence of the magnetic field is always zero.
磁場のフラックスは常にゼロである(磁気単極子が存在しないため)。
🐷磁場の発散は常にゼロである。
4. ファラデーの法則 (Faraday's Law of Induction)
The circulation of the electric field around a closed loop equals the negative time rate of change of the magnetic flux.
🐷The curl of the electric field is proportional to the negative time rate of change of the magnetic field.
閉曲線を回る電場の循環は、時間的に変化する磁場の負の時間変化率に等しい。
🐷電場の回転は、時間的に変化する磁場に比例する。
電磁波 (Electromagnetic Waves)
Electromagnetic waves consist of oscillating electric and magnetic fields propagating perpendicular to each other.
電場と磁場が互いに直交しながら伝播する波。
website: universe-review.ca
∇・E=ρがGauss's Law (Electric Field)
∇・B=0がGauss's Law (Magnetic Field)と呼ばれることもある。
【物理】量子力学(Quantum mechanics)
波動性と粒子性の二重性(Wave-Particle Duality)
Matter and light exhibit both particle-like properties (e.g., position, momentum) and wave-like properties (e.g., interference, diffraction).
物質や光は、粒子としての性質(例:位置、運動量)と波としての性質(例:干渉、回折)を同時に持つ。
例) 電子はスリットを通過するときに干渉縞を形成する(波動性)一方、検出器では個々の粒子として観測される(粒子性)。
シュレディンガー方程式 (Schrödinger Equation)
The Schrödinger equation describes the motion and energy of microscopic particles.
微小な粒子の運動とエネルギーを記述する波動方程式で、時間依存形は次のように表される。
不確定性原理 (Uncertainty Principle)
According to Heisenberg’s Uncertainty Principle, the position and momentum of a particle cannot be simultaneously measured with arbitrary precision
ハイゼンベルクの不確定性原理によると、粒子の位置と運動量は同時に正確に測定できない。
波動関数 (Wave Function)
The wave function φ(r, t) describes the probability distribution of a particle’s position and momentum. The squared magnitude represents the probability density of the particle’s position.
The state of a particle can be expressed as a superposition of multiple states.
波動関数 φ(r, t)は、粒子の位置や運動量の確率分布を記述し、波動関数の絶対値の2乗は、粒子が特定の位置に存在する確率密度を表す。
粒子の状態は複数の状態の重ね合わせとして表されることがある。
量子力学…もはや懐かしい…。
【地球物理】固体地球科学(Solid Earth)
この分野の論文は、英語でいつも読んでるのでメモ程度です。
プレートテクトニクス(Plate tectonics)
Driving Forces: Mantle convection, ridge push(mid-ocean ridges), and slab pull.
Boundary Types:
1. Divergent: Plates move apart (e.g., Mid-Atlantic Ridge).
2. Convergent: Plates collide, causing subduction or uplift (e.g., Himalayas).
3. Transform: Plates slide past each other (e.g., San Andreas Fault).
地殻(crust)
1. Continental crust
upper: granitic rocks, bottom: basaltic rocks, silica rich, 30-70km
2. Oceanic crust
basaltic rocks, denser, 5-10km
大陸地殻(上部:花崗岩質、下部:玄武岩質、シリカに富む)
海洋地殻(玄武岩質、密度高い)
マントル(Mantle)
-2850km mainly silicate minerals
Heat-driven flow in the mantle drives plate motion
1. Lithosphere
The rigit outer part of the Earth, including the crust and uppermost mantle.
2. Asthenosphere
A ductile region of the mantle where convection occurs, driving plate motion.
熱の移動によるマントル対流がプレート運動を駆動する。
核(Core)
primarily iron(Fe) and nickel(Ni)
1. Outer core
liquid state, generates the Earth’s magnetic field through convection
2. Inner core
solid state, due to immense pressure
【地球物理】地震学(Seismology)
地震波(Seismic waves)
1. P-waves (primary waves)
Longitudinal (compressional) waves.
Travel through solids, liquids, and gases.
2. S-waves (secondary waves)
Transverse (shear) waves.
Cannot propagate through liquids.
P波: 縦波であり、固体・液体・気体を伝播
S波: 横波であり、液体中では伝播できない
英語でのおすすめ学習ソース
数学
簡潔にまとめられたトピックごとの動画が見れます。
物理
このサイトの各トピックのEquation Overviewシリーズが非常に役に立ちました。例えば、電磁気だとCalculator Pad>Electromagnetism>Equation Overviewと、辿り着けます。
このサイトのContentsのTopic17に物理の基礎がトピックごとに見つけられます。理論だけではなくて現実での応用まで書かれていて読み物としても面白かった!
実際の面接
超絶緊張しました。
初めての英語での面接だったので…。
まず初めに、2人の教授が自己紹介と軽く専門分野について述べ、そのあと私の自己紹介。からの軽く雑談。
片方の教授がみっちりと質問リストを持っており、メールで予告された通り数学から始まりました。
最初の質問は、「常微分方程式(ordinary differential equations)と偏微分方程式(partial differential equations)はわかるよね?」みたいな確認から。
日本語では1回生レベルの質問でも、英語でこの科目をやってないってだけで1つ1つの数学用語にめちゃくちゃドキッとする😬
端的にODEsはsingle independent variableでPDEsはmultiple variableやからboundary and initial conditionsが必要、みたいなことを言った気がします。(緊張して覚えてへん)
そこからも、淡々と「超基礎的なことを自分の言葉で説明できるか」を試すような質問が続き、後半は「Why seismology is important to investigate the Earth's interior?」(地震学はなぜ地球の内部構造を調査するのに重要?)みたいな、広めな質問に。
私的には、こうゆう質問の方が今まで論文読んできたから話しやすかった!
あとは、「どのプログラミング言語でどんな解析をしたことがあるか」とかも聞かれました。
最後に、「卒業研究について説明して」と。
これこそ1週間ちゃんと練習してきた「待ってました!」の質問やったから、自分的によく説明できたと思う!
そして…面接官の2人が顔を見合わせ(Zoom上やから私の勝手な解釈)片方の教授が「We would like to welcome you to our institute😃」みたいな感じでその場で合格を頂きました!!嬉しすぎた!!!!
あとがき
基本は、
1. 科目ごとに抑えときたい概念をリストアップ
2. ChatGPT に日本語と英語で定義を生成してもらう(自分のノートと照らし合わせて理解&修正)
3. 自分で説明できるように何回も声に出して練習
の繰り返しをしてました。
その中でも、完全に忘れてる内容については、過去3年分のノートを総浚い。。
授業中のノートは、常にMicrosoftのOneNoteに取ってて、資料のPDFとかも毎回OneNote上に整理してました。
セメスター・授業ごとにまとめてて本当に良かった😮💨
クラウド上で保管しているので、留学中でも1年の1学期から振り返って勉強できました。見返す可能性のあるものはとにかくCloudに保管する!!!いつか役立つ!
この長すぎるnoteを一気読みする猛者はいないと思いますが(いたらコメントしてください)「あ、なんか1回生の時やったけど、これ何やったっけ…」って時に項目ごとに振り返ってもらえると思います。間違ってるところもあると思います(ごめんなさい)ので、指摘していただけるとありがたいです🙇♀️
以上!
いいなと思ったら応援しよう!

