2次曲線、主軸変換
連成振動を原島鮮「力学Ⅱ」に従って進めていたら、主軸変換という言葉が出てきた。なんのこっちゃウーロン茶である。主軸変換でググると行列の対角化がまた出てきた。さらに2次曲線。。 もう下流に戻れないのではと不安になりながら遡っていった。
1 2次曲線の確認
円、楕円、双曲線、放物線のことを2次曲線といい、
$${ax^2+bxy+cy^2+dx+ey+f=0}$$(1)の形で表現され、
$${b^2-4ac<0}$$のとき 楕円
$${b^2-4ac>0}$$のとき 双曲線
$${b^2-4ac=0}$$のとき 放物線 である。
1-1 放物線
定点(焦点$${\text{F}(p,0)}$$)と定直線(準線$${x=-p}$$)からの距離が等しい
点$${\text{P}(x, y)}$$の軌跡である。($${p\neq0}$$)
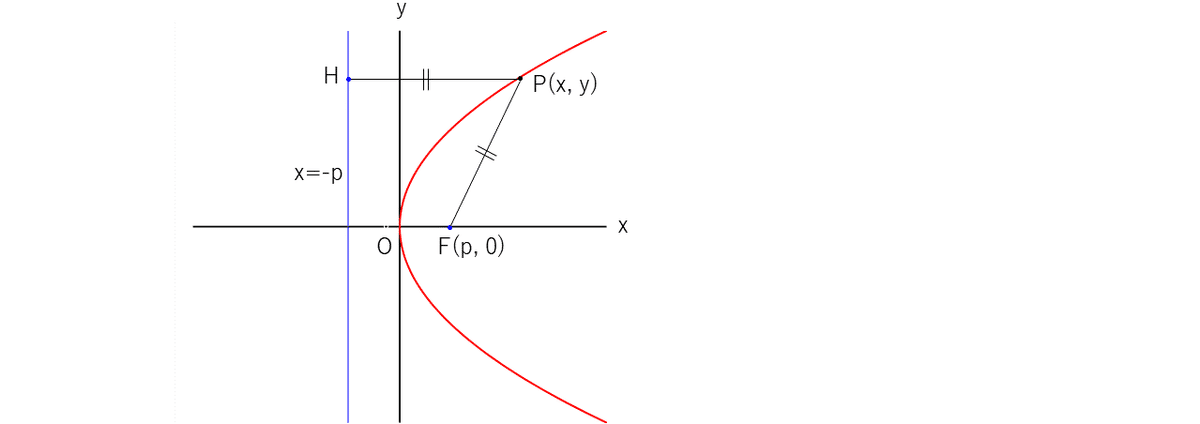
$${\overline{\text{PF}}=\overline{\text{PH}}}$$より $${\sqrt{(x-p)^2+y^2}=|x-(-p)|}$$
2乗して $${(x-p)^2+y^2=(x+p)^2}$$
$${x^2-2px+p^2+y^2=x^2+2px+p^2}$$
よって $${y^2=4px}$$ (2)(放物線の標準形)
または$${x}$$、$${y}$$を入れ替えて $${x^2=4py}$$ (3)(放物線の標準形2)
(2)は(1)において$${a=b=0}$$、(3)は$${b=c=0}$$なので
$${b^2-4ac=0}$$である。
1-2 楕円
2定点(焦点$${\text{F}(c,0), \text{F'}(-c,0)}$$)からの距離の和($${2a}$$とする)が等しい
点$${\text{P}(x, y)}$$の軌跡である。($${c\neq0, a>c>0}$$)
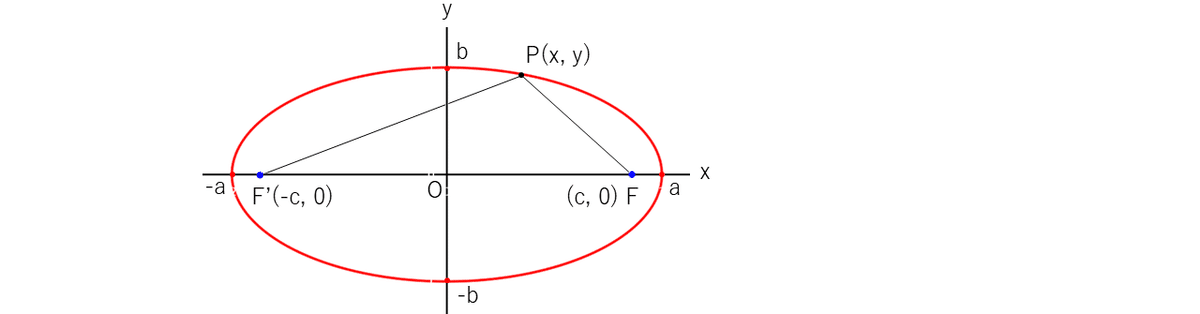
$${\overline{\text{PF}}+\overline{\text{PF'}}=2a}$$より $${\sqrt{(x-c)^2+y^2}+\sqrt{(x+c)^2+y^2}=2a}$$
$${\sqrt{(x-c)^2+y^2}=2a-\sqrt{(x+c)^2+y^2}}$$
2乗して
$${(x-c)^2+y^2=4a^2-4a\sqrt{(x+c)^2+y^2}+(x+c)^2+y^2}$$
$${x^2-2cx+c^2+y^2=4a^2-4a\sqrt{(x+c)^2+y^2}+x^2+2cx+c^2+y^2}$$
$${-2cx=4a^2-4a\sqrt{(x+c)^2+y^2}+2cx}$$
$${4a\sqrt{(x+c)^2+y^2}=4a^2+4cx}$$
$${a\sqrt{(x+c)^2+y^2}=a^2+cx}$$
2乗して
$${a^2x^2+2a^2cx+a^2c^2+a^2y^2=a^4+2a^2cx+c^2x^2}$$
$${a^2x^2+a^2c^2+a^2y^2=a^4+c^2x^2}$$ $${(a^2-c^2)x^2+a^2y^2=a^2(a^2-c^2)}$$
$${\dfrac{x^2}{a^2}+\dfrac{y^2}{a^2-c^2}=1}$$
$${a^2-c^2=b^2}$$とおくと $${\dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1}$$(4)(楕円の標準形)
(1)において $${a=\dfrac{1}{a'^2}, b=0, c=\dfrac{1}{b'^2}}$$なので
$${b^2-4ac=0-4×\dfrac{1}{a'^2}×\dfrac{1}{b'^2}<0}$$である。
1-3 双曲線
2定点(焦点$${\text{F}(c,0), \text{F'}(-c,0)}$$)からの距離の差($${2a}$$とする)が等しい
点$${\text{P}(x, y)}$$の軌跡である。($${c\neq0, a>c>0}$$)
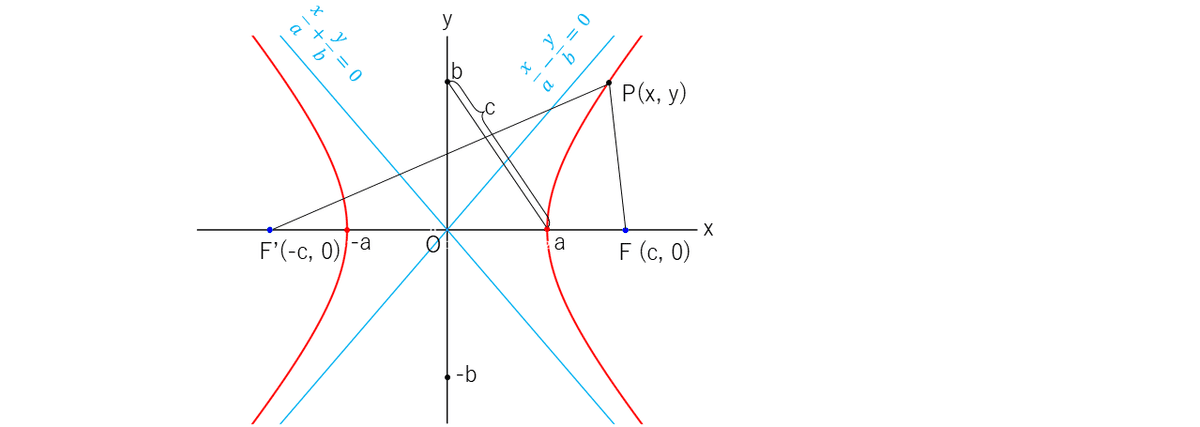
$${\overline{\text{PF}}-\overline{\text{PF'}}=\pm2a}$$より $${\sqrt{(x-c)^2+y^2}-\sqrt{(x+c)^2+y^2}=\pm2a}$$
$${\sqrt{(x-c)^2+y^2}=\pm2a+\sqrt{(x+c)^2+y^2}}$$
2乗して
$${(x-c)^2+y^2=4a^2\pm4a\sqrt{(x+c)^2+y^2}+(x+c)^2+y^2}$$
$${x^2-2cx+c^2+y^2=4a^2\pm4a\sqrt{(x+c)^2+y^2}+x^2+2cx+c^2+y^2}$$
$${\mp4a\sqrt{(x+c)^2+y^2}=4a^2+4cx}$$ $${\mp a\sqrt{(x+c)^2+y^2}=a^2+cx}$$
2乗して
$${a^2x^2+2a^2cx^2+a^2c^2+a^2y^2=a^4+2a^2cx+c^2x^2}$$
$${(a^2-c^2)x^2+a^2y^2=a^2(a^2-c^2)}$$
$${\dfrac{x^2}{a^2}+\dfrac{y^2}{a^2-c^2}=1}$$ $${\dfrac{x^2}{a^2}-\dfrac{y^2}{c^2-a^2}=1}$$
$${c^2-a^2=b^2}$$とおくと $${\dfrac{x^2}{a^2}-\dfrac{y^2}{b^2}=1}$$(5)(双曲線の標準形)
漸近線は $${\dfrac{x}{a}\pm\dfrac{y}{b}=0}$$である。
(1)において $${a=\dfrac{1}{a'^2}, b=0, c=-\dfrac{1}{b'^2}}$$なので
$${b^2-4ac=0-4×\dfrac{1}{a'^2}×\Big(-\dfrac{1}{b'^2}\Big)>0}$$である。
他にも平行移動、離心率、媒介変数表示などいくつか要があるが、ここで必要な前提ではない。以上ザクっと復習した。
2 主軸変換
2次曲線の一般形を以下のように変形する。(のちの変形の都合で$${b}$$は$${2b}$$とする。
$${ax^2+2bxy+cy^2+dx+ey+f=0}$$(1)(2次曲線の一般形)
$${ax^2+bxy+bxy+cy^2+dx+ey+f=0}$$
$${(ax+by)x+(bx+cy)y+dx+ey+f=0}$$
$${\begin{bmatrix}ax+by bx+cy\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+f=0}$$
$${\begin{bmatrix}x y\end{bmatrix}\begin{bmatrix}a b\\b c\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+f=0}$$ (6)
ここで$${\bm{A}=\begin{bmatrix}a b\\b c\end{bmatrix}}$$とする。
$${\bm{A}}$$に対する固有値:$${\lambda_1, \lambda_2}$$
固有ベクトル:$${\bm{x_1}, \bm{x_2}}$$(直交している)
規格化された固有ベクトル:$${\bm{u_1}, \bm{u_2}}$$( 〃 )
対角化ベクトル:$${\bm{P}=\begin{bmatrix}\bm{u_1} \bm{u_1}\end{bmatrix}}$$(規格化されている)
$${\bm{P}}$$の逆ベクトル:$${\bm{P}^{-1}}$$
$${\bm{P}}$$の転置ベクトル:$${{^t\bm{P}}}$$($${{^t\bm{P}}=\bm{P}^{-1}}$$である)
とする。
以上より
$${\begin{bmatrix}x\\y\end{bmatrix}=\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}}$$(7)、また$${\begin{bmatrix}x'\\y'\end{bmatrix}={^t\bm{P}}\begin{bmatrix}x\\y\end{bmatrix}}$$(8)、$${^t\bm{P}\bm{A}\bm{P}=\begin{bmatrix}\lambda_1 0\\0 \lambda_2\end{bmatrix}}$$(9)
では、変形をはじめる
$${\begin{bmatrix}x y\end{bmatrix}\begin{bmatrix}a b\\b c\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+f=0}$$ (6)
$${\begin{matrix}\\ \end{matrix}^t\begin{bmatrix}x\\ y\end{bmatrix}\bm{A}\begin{bmatrix}x\\y\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+f=0}$$
(7)を代入
$${\begin{matrix}\\ \end{matrix}^t\bigg\{\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}\bigg\}\bm{A}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+f=0}$$
$${\begin{matrix}\\ \end{matrix}^t\begin{bmatrix}x'\\y'\end{bmatrix}{^t\bm{P}}\bm{A}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+f=0}$$
(9)を代入
$${\begin{bmatrix}x' y'\end{bmatrix}\begin{bmatrix}\lambda_1 0\\0 \lambda_2\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+f=0}$$
$${\begin{bmatrix}\lambda_1x' \lambda_2y'\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+\begin{bmatrix}d e\end{bmatrix}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+f=0}$$
$${\begin{bmatrix}d e\end{bmatrix}\bm{P}=\begin{bmatrix}d' e'\end{bmatrix}}$$とする
$${\begin{bmatrix}\lambda_1x' \lambda_2y'\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+\begin{bmatrix}d' e'\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+f=0}$$
$${\lambda_1x'^2+\lambda_2y'^2+d'x'+e'y'+f=0}$$ (11)($${x'y'}$$の項が無くなった!)
ここで座標系$${\text{Oxy}}$$と座標系$${\text{Ox'y'}}$$の関係を考える。
座標系$${\text{Ox'y'}}$$は座標系$${\text{Oxy}}$$を原点中心に半時計まわりに$${\theta}$$回転したものすると
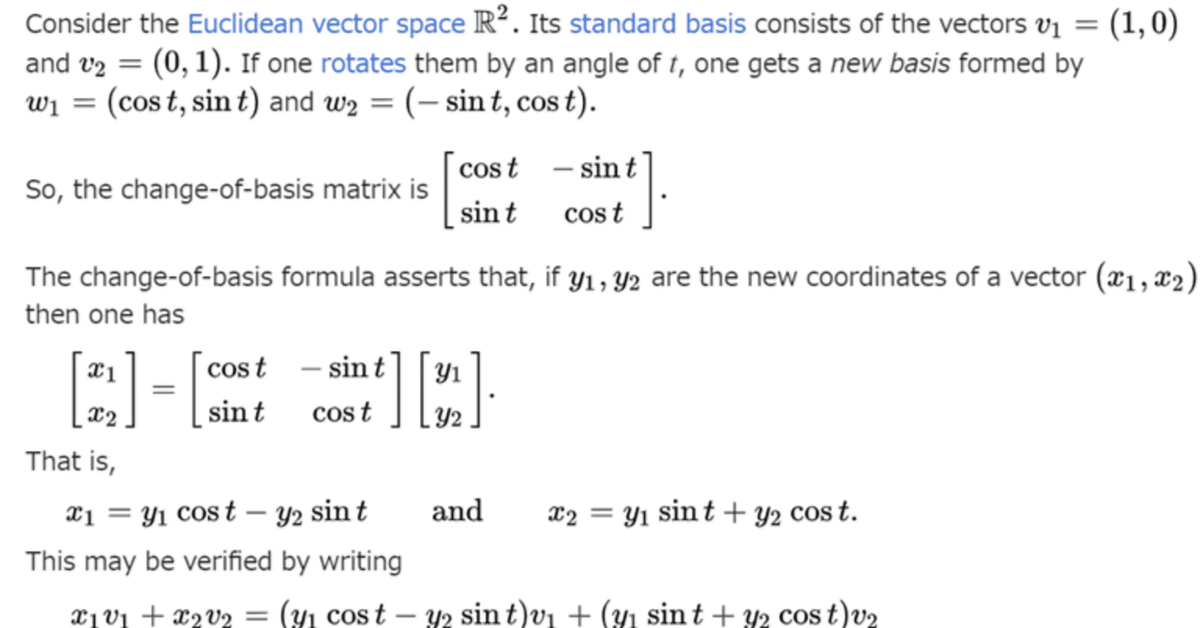
$${\begin{bmatrix}x'\\y'\end{bmatrix}=\begin{bmatrix}\cos\theta \sin\theta\\-\sin\theta \cos\theta\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}}$$
よって
$${\begin{bmatrix}x\\y\end{bmatrix}=\begin{bmatrix}\cos\theta \sin\theta\\-\sin\theta \cos\theta\end{bmatrix}^{-1}\begin{bmatrix}x'\\y'\end{bmatrix}=\begin{bmatrix}\cos\theta -\sin\theta\\\sin\theta \cos\theta\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}}$$
$${=\begin{bmatrix}x'\cos\theta-y'\sin\theta\\x'\sin\theta+y' \cos\theta\end{bmatrix}}$$(12)
(7)と比べると
$${\bm{P}=\begin{bmatrix}\cos\theta -\sin\theta\\\sin\theta \cos\theta\end{bmatrix}}$$(13)である。
(1)に(12)を代入する
$${ax^2+bxy+cy^2+dx+ey+f=0}$$(1)
$${a(x'\cos\theta-y'\sin\theta)^2+b(x'\cos\theta-y'\sin\theta)(x'\sin\theta+y' \cos\theta)}$$
$${+c(x'\sin\theta+y' \cos\theta)^2+d(x'\cos\theta-y'\sin\theta)}$$
$${+e(x'\sin\theta+y' \cos\theta)+f=0}$$
$${ax'^2\cos^2\theta-2ax'y'\sin\theta\cos\theta+ay'^2\sin^2\theta+bx'^2\sin\theta\cos\theta}$$
$${+(b\cos^2\theta-b\sin^2\theta)x'y'-by'^2\sin\theta\cos\theta+cx'^2\sin^2\theta}$$
$${+2cx'y'\sin\theta\cos\theta+cy'^2\cos^2\theta+dx'\cos\theta-dy'\sin\theta}$$
$${+ex'\sin\theta+ey' \cos\theta+f=0}$$
$${(a\cos^2\theta+b\sin\theta\cos\theta+c\sin^2\theta)x'^2}$$
$${+\{2(c-a)\sin\theta\cos\theta+b(\cos^2\theta-\sin^2\theta)\}x'y'}$$
$${+(a\sin^2\theta-b\sin\theta\cos\theta+c\cos^2\theta)y'^2+(d\cos\theta+e\sin\theta)x'}$$
$${+(-d\sin\theta+e\cos\theta)y'+f=0}$$
(11)は$${x'y'}$$の係数=0なので
$${2(c-a)\sin\theta\cos\theta+b(\cos^2\theta-\sin^2\theta)=0}$$
$${2(c-a)\dfrac{1}{2}\sin(\theta+\theta)+b\cos(\theta+\theta)=0}$$
$${(c-a)\sin2\theta+b\cos2\theta=0}$$ (14)
$${\dfrac{\sin2\theta}{\cos2\theta}=\tan2\theta=\dfrac{b}{a-c}}$$ $${\theta=\dfrac{1}{2}\tan^{-1}\Big(\dfrac{b}{a-c}\Big)}$$ (15)
(i) $${a=c}$$のとき (14)より$${b\cos2\theta=0}$$ $${2\theta=\pm\dfrac{\pi}{2}}$$ $${\theta=\pm\dfrac{\pi}{4}}$$
(ii) $${a\neq c}$$のとき (15)より$${\theta=\dfrac{1}{2}\tan^{-1}\Big(\dfrac{b}{a-c}\Big)}$$
以上をまとめると
座標系Oxy $${ax^2+bxy+cy^2+dx+ey+f=0}$$(1)
↓ 半時計方向に$${\theta}$$回転
↓ $${\begin{bmatrix}x'\\y'\end{bmatrix}=\begin{bmatrix} \cos\theta \sin\theta\\-\sin\theta \cos\theta\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}=\bm{P}^{-1}\begin{bmatrix}x\\y\end{bmatrix}}$$
座標系Ox'y' $${\lambda_1x'^2+\lambda_2y'^2+d'x'+e'y'+f=0}$$(11)
3 具体例
3-1 その1
$${5x^2-6xy+5y^2-16\sqrt{2}x+16\sqrt{2}y+24=0}$$ (15)
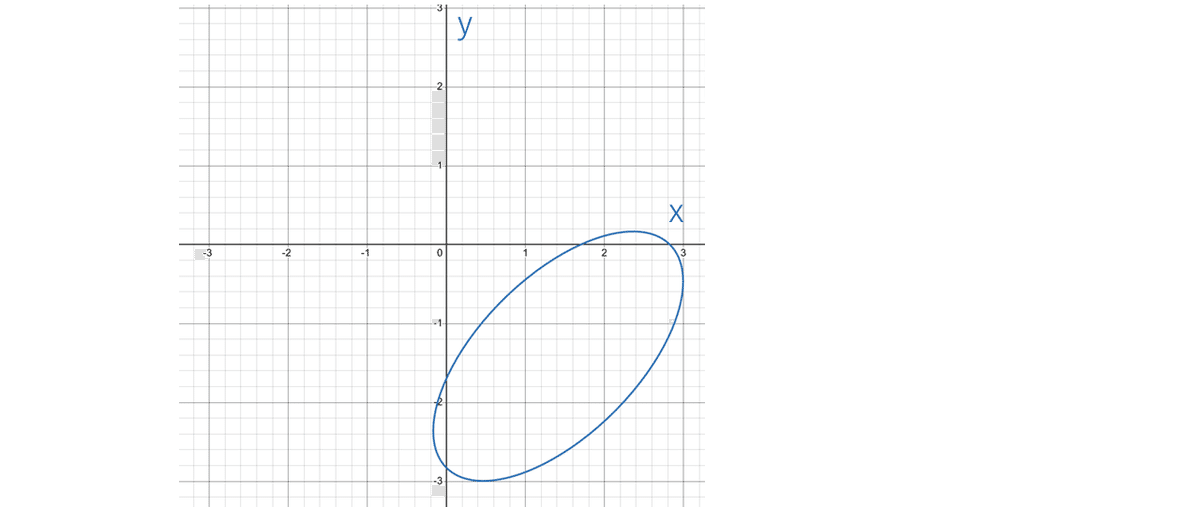
(15)を変形
$${\begin{bmatrix}x y\end{bmatrix}\begin{bmatrix}5 3\\3 5\end{bmatrix}\begin{bmatrix}x\\y\end{bmatrix}+16\sqrt{2}(-1 1)\begin{bmatrix}x\\y\end{bmatrix}+24=0}$$ (16)
$${\bm{A}=\begin{bmatrix}5 3\\3 5\end{bmatrix}}$$
$${\Big(\begin{bmatrix}5 3\\3 5\end{bmatrix}-\lambda\bm{E}\Big)\bm{x}=\begin{bmatrix}5-\lambda 3\\3 5-\lambda\end{bmatrix}\bm{x}=0}$$ (17)
$${(5-\lambda)^2-3^3=0 (2-\lambda)(8-\lambda)=0 \lambda_1=2, \lambda_1=8}$$
$${\lambda=2(\lambda_1)}$$のとき
$${\begin{bmatrix}5-2 3\\3 5-2\end{bmatrix}\begin{bmatrix}a_1\\a_2\end{bmatrix}=0 3a_1+3a_2=0}$$
$${\bm{x_1}=k_1\begin{bmatrix}1\\1\end{bmatrix} \bm{u_1}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1\\1\end{bmatrix}}$$
$${\lambda=8(\lambda_2)}$$のとき
$${\begin{bmatrix}5-8 3\\3 5-8\end{bmatrix}\begin{bmatrix}b_1\\b_2\end{bmatrix}=0 -3a_1+3a_2=0}$$
$${\bm{x_2}=k_2\begin{bmatrix}-1\\1\end{bmatrix} \bm{u_2}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}-1\\1\end{bmatrix}}$$
よって
$${\bm{P}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1 -1\\1 1\end{bmatrix} ^t\bm{P}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1 1\\1 -1\end{bmatrix}}$$
※ $${\bm{u_2}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1\\-1\end{bmatrix}}$$も理屈ではありだが、このとき$${\bm{P}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1 1\\1 -1\end{bmatrix}}$$
$${=\begin{bmatrix}\cos\theta -\sin\theta\\\sin\theta \cos\theta\end{bmatrix}}$$を満たす$${\theta}$$がない。対角成分($${\cos\theta}$$)は等しく
なければならない)
$${\begin{bmatrix}x\\y\end{bmatrix}=\bm{p}\begin{bmatrix}x'\\y'\end{bmatrix}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1 -1\\1 1\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}=\begin{bmatrix}\cos(\pi/4) -\sin(\pi/4)\\\sin(\pi/4) \cos(\pi/4)\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}}$$
以上を(16)に代入
$${{^t\begin{bmatrix}x\\y\end{bmatrix}}\bm{A}\begin{bmatrix}x\\y\end{bmatrix}+16\sqrt{2}(-1 1)\begin{bmatrix}x\\y\end{bmatrix}+24=0}$$
$${{^t\Big\{\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}}\Big\}\bm{A}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+16\sqrt{2}(-1 1)\dfrac{1}{\sqrt{2}}\begin{bmatrix}1 -1\\1 1\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+24=0}$$
$${{^t\begin{bmatrix}x'\\y'\end{bmatrix}}{^t\bm{P}}\bm{A}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+16(-1+1 1+1)\begin{bmatrix}x'\\y'\end{bmatrix}+24=0}$$
$${{\begin{bmatrix}x' y'\end{bmatrix}}\begin{bmatrix}2 0\\0 8\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+16(-1+1 1+1)\begin{bmatrix}x'\\y'\end{bmatrix}+24=0}$$
$${\begin{bmatrix}2x' 8y'\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+16×2y'+24=0}$$
$${2x'^2+8y'^2+32y'+24=0}$$
$${x'^2+4y'^2+16y'+12=0}$$
$${x'^2+4(y'+2)^2-16+12=0}$$
$${x'^2+4(y'+2)^2=4}$$
$${\dfrac{x'^2}{2^2}+(y'+2)^2=1}$$
新しい主軸系Ox'y'(座標系Oxyを反時計まわりに$${\pi/4}$$回転)で(0, -2)を中心とする長径(x'軸方向)2、短径(y'軸方向)1の楕円である。
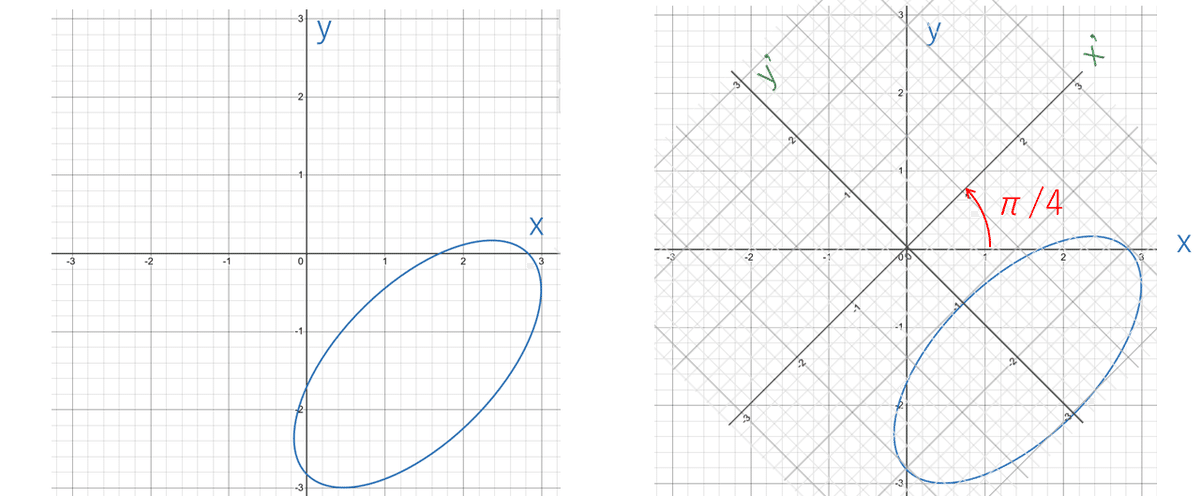
上では$${\bm{P}=\begin{bmatrix}\bm{u_1 u_2}\end{bmatrix}}$$としたが、$${\bm{u_1}}$$、$${\bm{u_2}}$$の順序を入れ替えてみよう。理屈ではありである。
$${\bm{P}=\begin{bmatrix}\bm{u_2 u_1}\end{bmatrix}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}-1 1\\ 1 1\end{bmatrix}}$$となるが、対角成分($${\cos\theta}$$)が等しくない。そこで$${\bm{u_2}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}-1\\1\end{bmatrix}}$$を$${\bm{u_2}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1\\-1\end{bmatrix}}$$としよう。
$${\bm{P}=\dfrac{1}{\sqrt{2}}\begin{bmatrix} 1 1\\-1 1\end{bmatrix}}$$となる。 $${^t\bm{P}=\dfrac{1}{\sqrt{2}}\begin{bmatrix}1 -1\\1 1\end{bmatrix}}$$ $${^t\bm{PAP}=\begin{bmatrix}8 0\\0 2\end{bmatrix}}$$
$${\begin{bmatrix}x\\y\end{bmatrix}=\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}=\dfrac{1}{\sqrt{2}}\begin{bmatrix} 1 1\\-1 1\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}=\begin{bmatrix}\cos(-\pi/4) -\sin(-\pi/4)\\\sin(-\pi/4) \cos(-\pi/4)\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}}$$
これらを(16)に代入
$${{^t\begin{bmatrix}x\\y\end{bmatrix}}\bm{A}\begin{bmatrix}x\\y\end{bmatrix}+16\sqrt{2}(-1 1)\begin{bmatrix}x\\y\end{bmatrix}+24=0}$$
$${{^t\Big\{\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}}\Big\}\bm{A}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+16\sqrt{2}(-1 1)\dfrac{1}{\sqrt{2}}\begin{bmatrix} 1 1\\-1 1\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+24=0}$$
$${{^t\begin{bmatrix}x'\\y'\end{bmatrix}}{^t\bm{P}}\bm{A}\bm{P}\begin{bmatrix}x'\\y'\end{bmatrix}+16(-1-1 -1+1)\begin{bmatrix}x'\\y'\end{bmatrix}+24=0}$$
$${{\begin{bmatrix}x' y'\end{bmatrix}}\begin{bmatrix}8 0\\0 2\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+16(-2 0)\begin{bmatrix}x'\\y'\end{bmatrix}+24=0}$$
$${\begin{bmatrix}8x' 2y'\end{bmatrix}\begin{bmatrix}x'\\y'\end{bmatrix}+16×(-2x')+24=0}$$
$${8x'^2+2y'^2-32x'+24=0}$$
$${4x'^2+y'^2-16x'+12=0}$$
$${4(x'^2-4x'+4)-16+y'^2+12=0}$$
$${4(x'-2)^2+y'^2=4}$$
$${(x'-2)^2+\dfrac{(y'+2)^2}{2^2}=1}$$
新しい主軸系Ox'y'(座標系Oxyを時計まわりに$${\pi/4}$$回転)で(2, 0)を中心とする長径(y'軸方向)2、短径(x'軸方向)1の楕円である。
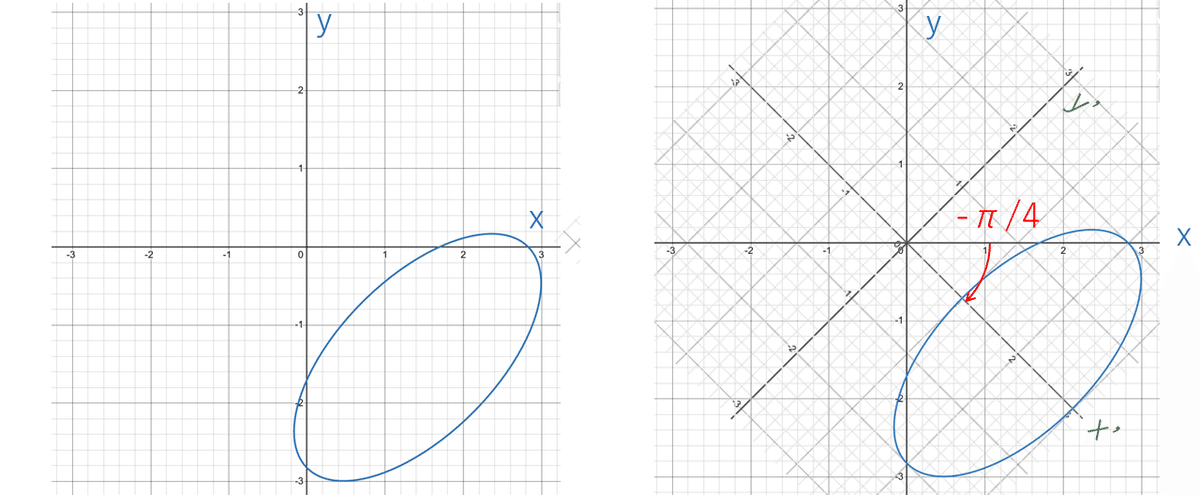
以上
双曲線、放物線についても具体例をあげるつもりだったが疲れた。まあこれでよしとしよう。
取りあえず源流のいけるところまでいったということで下流に戻ろう。下流に大きな深みがないことを祈る。あっぷっぷ
追記
グラフの描画にはじめエクセルを使ったが、媒介変数を使ってx、yを出して散布図にした。面倒なので iPadのアプリを探したらf(x, y)=0をそのまま入力したら描いてくれる便利なものが見つかった。どうやってx ,yを求めているのか、まさか裏で主軸変換していることはないだろうがアルゴリズムに興味が湧く。そのうち調べたい。使ったのは↓のiPad版。便利な世の中になった。
